Program
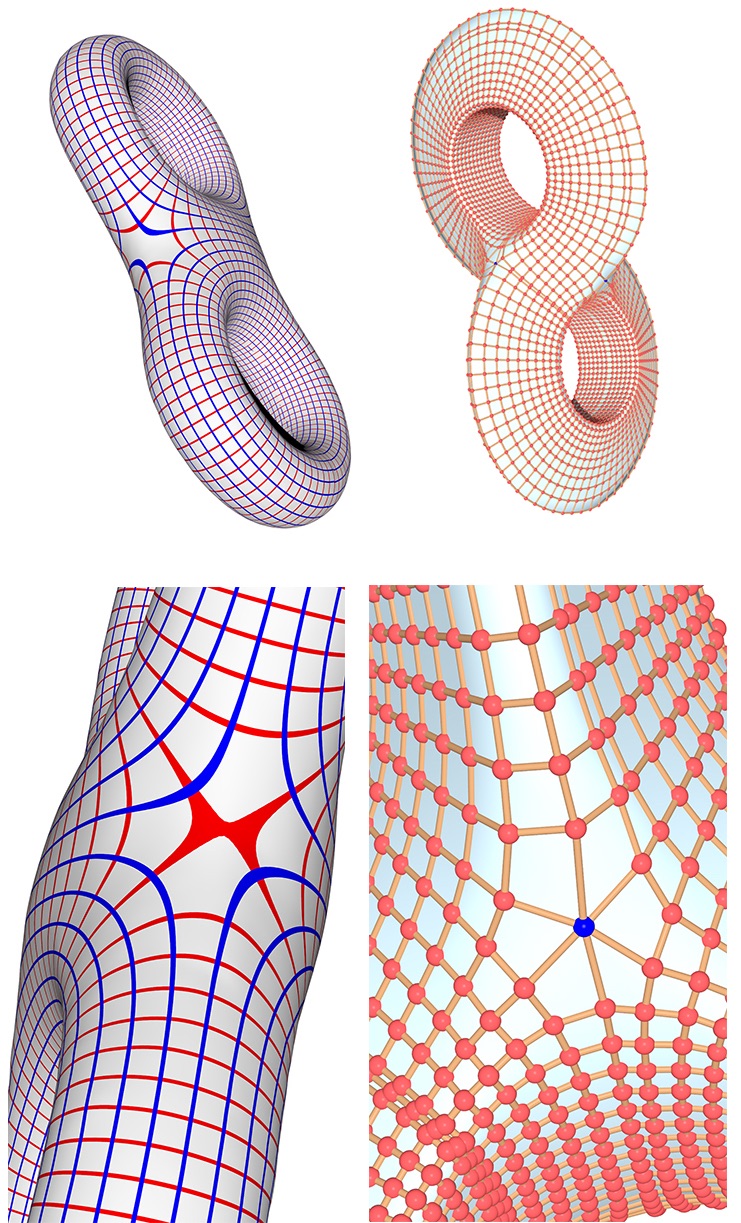
Some holomorphic differentials on a genus 2 surface, with close up views of singular points, image courtesy Jian Jiang.
Holomorphic differentials on Riemann surfaces have long held a distinguished place in low dimensional geometry, dynamics and representation theory. Recently it has become apparent that they constitute a common feature of several other highly active areas of current research in mathematics and also at the interface with physics. In some cases the areas themselves (such as stability conditions on Fukaya-type categories, links to quantum integrable systems, or the physically derived construction of so-called spectral networks) are new, while in others the novelty lies more in the role of the holomorphic differentials (for example in the study of billiards in polygons, special - Hitchin or higher Teichmuller - components of representation varieties, asymptotic properties of Higgs bundle moduli spaces, or in new interactions with algebraic geometry).
It is remarkable how widely scattered are the motivating questions in these areas, and how diverse are the backgrounds of the researchers pursuing them. Bringing together experts in this wide variety of fields to explore common interests and discover unexpected connections is the main goal of our program. Our program will be of interest to those working in many different elds, including low-dimensional dynamical systems (via the connection to billiards); differential geometry (Higgs bundles and related moduli spaces); and different types of theoretical physics (electron transport and supersymmetric quantum field theory).
Bibliography
Preprints
- Masur-Veech volumes, frequencies of simple closed geodesics and intersection numbers of moduli spaces of curves
- The vanishing rate of Weil-Petersson sectional curvatures
- Higgs bundles -- Recent applications
- Orthogonal Higgs bundles with singular spectral curves
- What if Planet 9 is a Primordial Black Hole?
- Ultraviolet Freeze-in and Non-Standard Cosmologies
- Exponential BPS graphs and D brane counting on toric Calabi-Yau threefolds: Part I
- Multi-cover skeins, quivers, and 3d \mathcal{N}=2 dualities
- Higgs bundles without geometry
- Minimal percolating sets for mutating infectious diseases
- Reviving Z and Higgs Mediated Dark Matter Models in Matter Dominated Freeze-out
- Generalized B-Opers
- Stability conditions, cluster varieties, and Riemann-Hilbert problems from surfaces
Keywords and Mathematics Subject Classification (MSC)
Tags/Keywords
holomorphic differentials
spectral networks
Higgs bundles
wall-crossing
stability conditions
| August 15, 2019 - August 16, 2019 | Connections for Women: Holomorphic Differentials in Mathematics and Physics |
| August 19, 2019 - August 23, 2019 | Introductory Workshop: Holomorphic Differentials in Mathematics and Physics |
| November 18, 2019 - November 22, 2019 | Holomorphic Differentials in Mathematics and Physics |