Home  /  Complex dynamics: from special families to natural generalizations in one and several variables

Program

Complex Dynamics: from special families to natural generalizations in one and several variables January 18, 2022 to May 27, 2022
Organizers LEAD Sarah Koch (University of Michigan), Jasmin Raissy (Institut de Mathématiques de Bordeaux), Dierk Schleicher (Université d'Aix-Marseille (AMU)), Mitsuhiro Shishikura (Kyoto University), Dylan Thurston (Indiana University)
Description
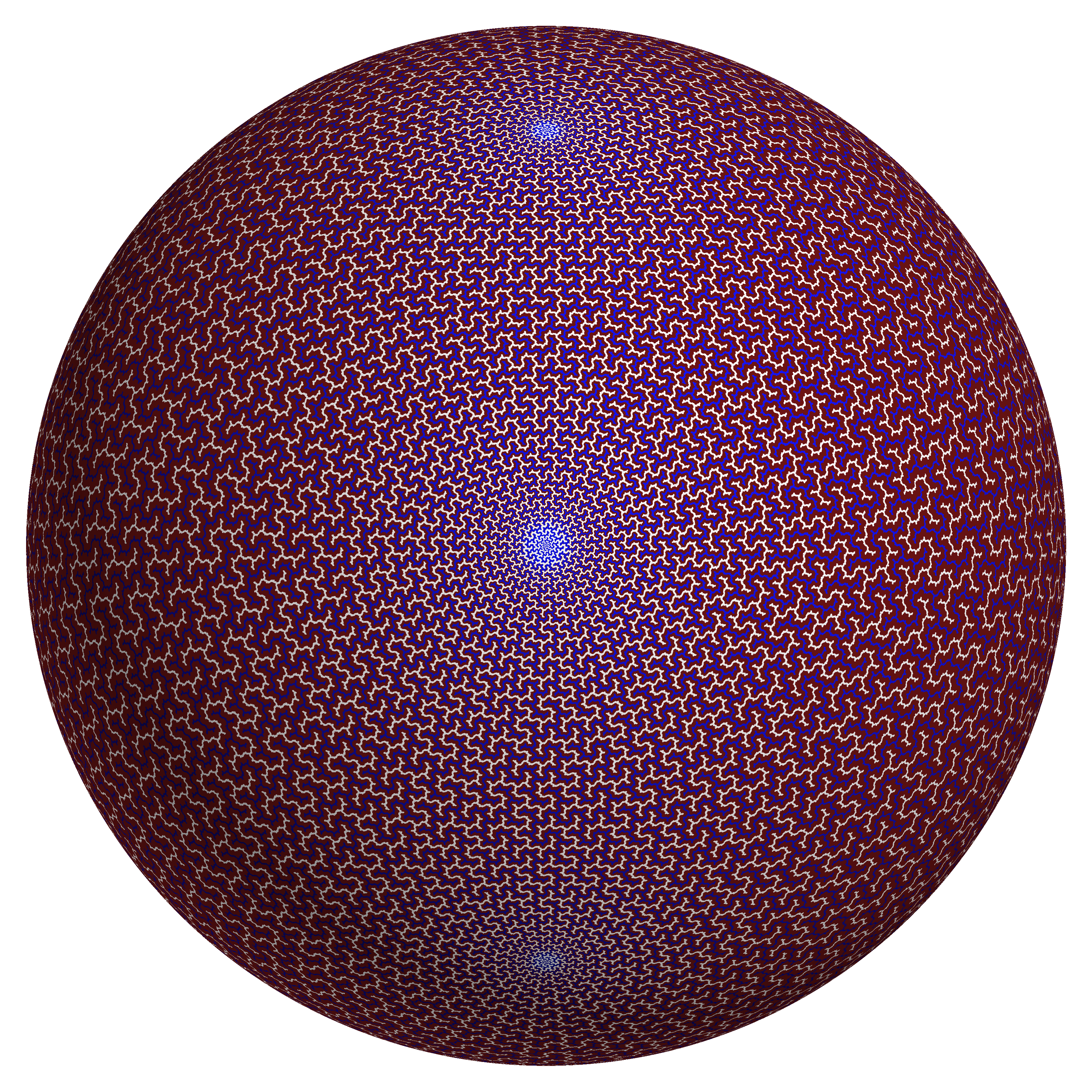
Image
The mating of these two dendritic Julia sets is equal to the Julia set of a rational map of degree 2; that Julia set is equal to the entire Riemann sphere. Picture by Arnaud Chéritat
Holomorphic dynamics is a vibrant field of mathematics that has seen profound progress over the past 40 years. It has numerous interconnections to other fields of mathematics and beyond.  Our semester will focus on three selected classes of dynamical systems: rational maps (postcritically finite and beyond); transcendental maps; and maps in several complex variables. We will put particular emphasis on the interactions between each these, and on connections with adjacent areas of mathematics.  Bibliography
Keywords and Mathematics Subject Classification (MSC)
Tags/Keywords

  • Holomorphic dynamics

  • iteration

  • Fatou set

  • Julia set

  • Mandelbrot set

  • renormalization

  • parameter spaces

  • postcritically finite maps

  • iterated monodromy groups

  • Thurston theory

  • arithmetic dynamics

  • transcendental dynamics

  • quasiconformal folding

  • Several Complex Variables

  • wandering domains

Primary Mathematics Subject Classification
Secondary Mathematics Subject Classification No Secondary AMS MSC
Logistics Program Logistics can be viewed by Members. If you are a program member then Login Here.
Programmatic Workshops
February 02, 2022 - February 04, 2022 [HYBRID WORKSHOP] Connections Workshop: Complex Dynamics - from special families to natural generalizations in one and several variables
February 08, 2022 - February 17, 2022 [HYBRID WORKSHOP] Introductory Workshop: Complex Dynamics - from special families to natural generalizations in one and several variables
May 02, 2022 - May 06, 2022 Adventurous Berkeley Complex Dynamics