Program
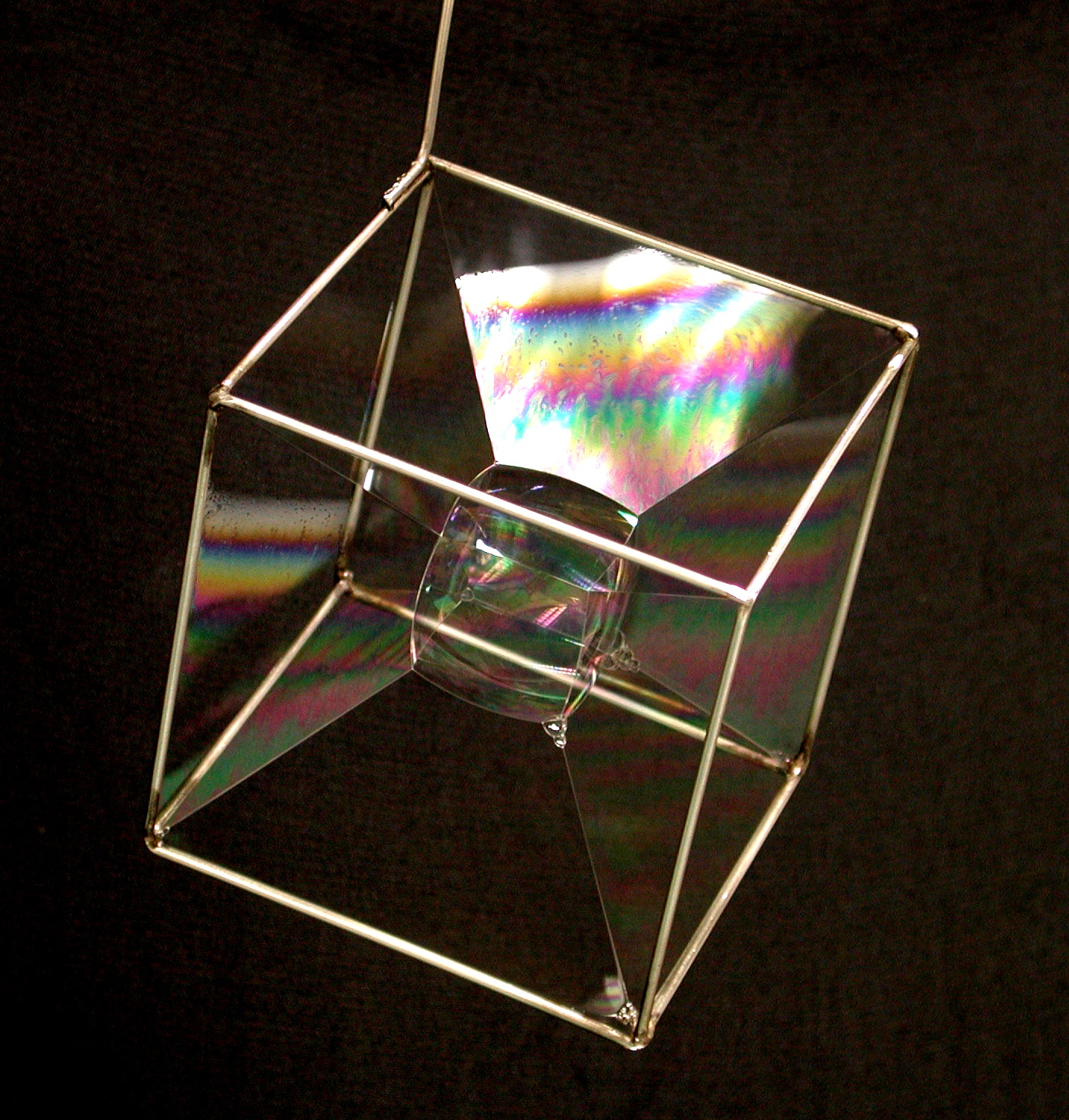
“Plateau’s Memory ” (by A. van der Net): A soap film with singularities
This program sits at the intersection between differential geometry and analysis but also connects to several other adjacent mathematical fields and to theoretical physics. Differential geometry aims to answer questions about very regular geometric objects (smooth Riemannian manifolds) using the tools of differential calculus. A fundamental object is the curvature tensor of a Riemannian metric: an algebraically complicated object that involves 2nd partial derivatives of the metric. Many questions in differential geometry can therefore be translated into questions about the existence or properties of the solutions of systems of (often) nonlinear partial differential equations (PDEs). The PDE systems that arise in geometry have historically stimulated the development of powerful new analytic methods. In most cases the nonlinearity of these systems makes ‘closed form’ expressions for a solution impossible: instead more abstract methods must be employed.
The aim of this program is to study geometric and analytic aspects of special holonomy metrics, instanton bundle over such spaces and the calibrated submanifolds within them. Because these objects are Einstein metrics, Yang–Mills connections and minimal submanifolds respectively they fit into a wider geometric framework. But because each of them satisfies a first-order PDE system they enjoy various special properties. This program takes the position that the natural viewpoint, both geometrically and analytically, is to consider these three classes of closely-related geometric objects together. A common feature is that weak solutions to these higher-dimensional equations may have interesting non-isolated singular sets whose detailed geometric and analytic structure is still poorly understood. Improving our understanding of such (partial) regularity of solutions is a key component of the program.
Keywords and Mathematics Subject Classification (MSC)
Tags/Keywords
Calabi–Yau
G2
Spin(7)
Collapsing
Exceptional holonomy
Calibrations
Instantons
Tangent Cones
Microlocal analysis
Generic Singularities
Lagrangian Mean curvature flow
G2 Laplacian flow
Pluripotential Theory
Regularity theory
singularity formation
Kähler–Einstein
Kähler–Ricci flow
Hyperkähler
14J32 - Calabi-Yau manifolds (algebro-geometric aspects) [See also 32Q25]
53C26 - Hyper-Kähler and quaternionic Kähler geometry, "special'' geometry
53C55 - Global differential geometry of Hermitian and Kählerian manifolds [See also 32Qxx]
53E20 - Ricci flows [See also 53Exx, 53E40, 53E50, 53E99, 53E30, 53E10]
58J40 - Pseudodifferential and Fourier integral operators on manifolds [See also 35Sxx]
| August 21, 2024 - August 23, 2024 | Connections Workshop: New Frontiers in Curvature & Special Geometric Structures and Analysis |
| September 03, 2024 - September 06, 2024 | Introductory Workshop: Special Geometric Structures and Analysis |
| November 18, 2024 - November 22, 2024 | Geometry and analysis of special structures on manifolds |