Summer Graduate School

<p>A display of the evolution of an Erdos-Renyi random graph .&nbsp;</p>

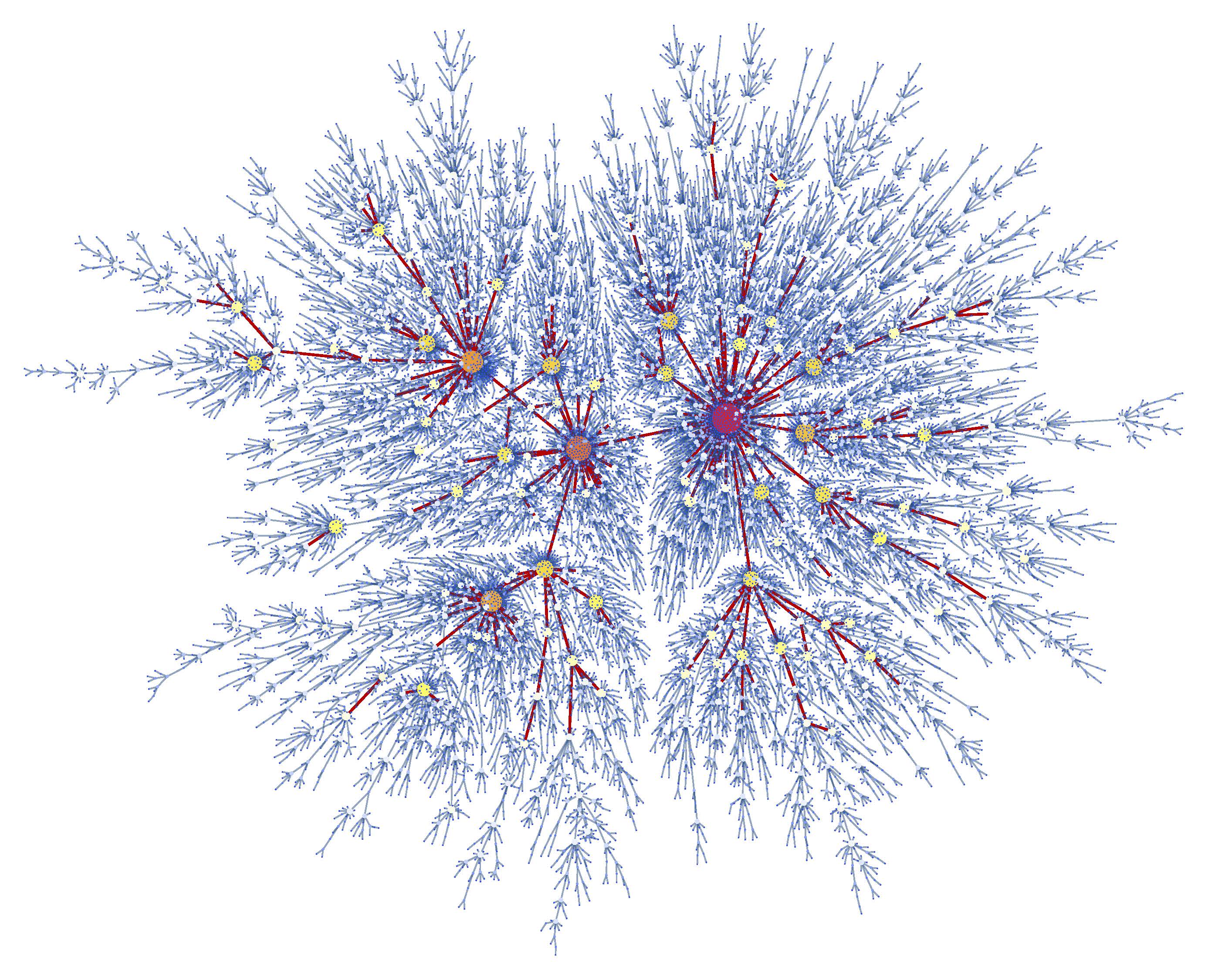
Random graphs are ubiquitous in modern probability theory. Besides their intrinsic mathematical beauty, they are also used to model complex networks. In the early 2000’s, I. Benjamini and O. Schramm introduced a mathematical framework in which they endowed the set of locally finite rooted connected graphs with the structure of a Polish space, called the local topology. The goal of this summer school is to introduce the framework of local limits of random graphs, the concepts of Benjamini-Schramm (or unbiased) limits and unimodularity, as well as the most important applications. The lectures will be delivered by Nicolas Curien (Prof. Paris-Saclay University) and Justin Salez (Prof. Université Paris-Dauphine) and will be complemented by many problem sessions, where students will work in small groups under the guidance of teaching assistants, who are researchers in the field. 

School Structure

The school will be comprised of two lectures and two problem sessions each day. Students will also have the opportunity for informal discussions areound food and drinks at the daily evening sessions, when some research talks will be given by senior researchers in the field.

Prerequisites

In probability, students should have a good understanding of the following topics, usually covered in any graduate course in (discrete) probability theory: convergence of measures (convergence in law in Polish spaces), Poisson processes, countable Markov chains, martingales (convergence theorems), and basic notions in statistics. Standard references on these topics are Jean-François Le Gall’s « Measure Theory, Probability, and Stochastic Processes », chapter 1 of Patrick Bilingsley’s « Convergence of Probability Measures », or Rick Durrett’s « Probability: Theory and Examples». 

Ideally, the students should also have learned some graph theory, discrete potential theory (random walks on graphs), and ergodic theory. A little familiarity with the most important random graph models such as Erdös-Rényi graphs or Galton—Watson trees would also be helpful (see for example Nicolas Curien’s lecture notes « Random Graphs, quodlibet », available here). However, the prerequisites listed in this paragraph are not mandatory, as the most relevant results from these areas will be reviewed during the lectures.

Application Procedure

SLMath is only able to support a limited number of students to attend this school.  Therefore, it is likely that only one student per institution will be funded by SLMath.

For eligibility and how to apply, see the Summer Graduate Schools homepage.

Venue

The summer school will take place at Orsay’s Mathematics Institute located on the campus of Paris-Saclay University (about 35km in the south of Paris). The students will be housed on campus in the Rives de l’Yvette dormitory.