Summer Graduate School

From micro to macro, sea ice supports life in the polar regions. Left: illustration of the porous sea ice microstructure, home to a microbial community of ice endemic organisms including bacteria and algae. Right: two penguins on Antarctic sea ice.

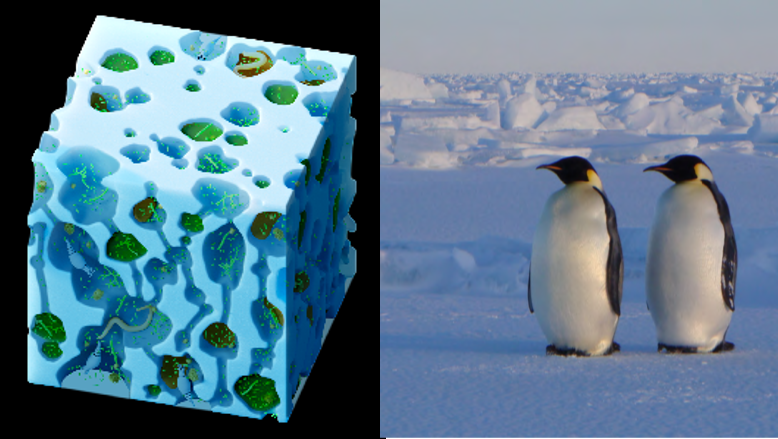
In this summer school, students will be introduced to mathematical and computational modeling of sea ice and polar ecosystems in a warming climate. As a material, sea ice is a multiscale composite structured on length scales ranging from tenths of millimeters to tens of kilometers. From tiny brine inclusions and surface melt ponds of increasing complexity, to ice floes of varying sizes in a seawater host, a principal challenge is how to find sea ice effective properties that are relevant to larger scale climate and process models, given data on smaller scale structures. Similarly, the sea ice ecosystem ranges from algae living in the brine inclusions to charismatic megafauna like penguins and polar bears, whose diets depend critically, down the line, on the tiny sea ice extremophiles. The dynamics of sea ice microbial communities are regulated by the physics of the ice microstructure, and, in turn, many of these microbes modify their environment by secreting extracellular polymeric substances. In addition to sea ice and its ecosystems, we will consider mathematical modeling of the broader climate system, including energy balance models, climate and ecological tipping points, and global circulation models.

School Structure

The school will consiste of two lectures and two collaborative sessions each day.

Prerequisites

In order to facilitate the nomination process, we have divided our prerequisites list into three broad categories: “core” or “essential” topics that are basic, minimal requirements for a student to succeed in the summer school; “recommended” topics that not all students would have had, but that would be beneficial; and “nice to have” or “wish list” topics that perhaps only a few of the attendees will have seen. Within each category below, topics are listed in roughly descending order of priority. No student should be nominated that lacks a background in the “core” or “essential” areas. Students having coursework in at least two or three of the “recommended” areas should be considered strong candidates. Students having exposure to several of the “nice to have” or “wish list” areas will be well-positioned for a very enriching experience and will likely be at ease with much of the introductory material. We emphasize that a lack of “recommended” or “nice to have” background topics should not be disqualifying for otherwise strong students; as the organizers have many years of experience successfully introducing junior academics to various topics on an “as-needed” basis. Indeed, a major goal of the summer school will be to develop the basic knowledge necessary to address current research topics in the field.

1.    Core/Essential 
(a)     Undergraduate real analysis at the level of Rudin, Principles of Mathematical Analysis, Chapters 1-8, 11 
(b)     Undergraduate linear algebra at the level of Lay, Linear Algebra and its Applications, 5th ed., Chapters 1-7
(c)     Undergraduate ODE at the level of Boyce and DiPrima, Elementary Differential Equations and Boundary Value Problems, 11th ed., Chapters 1-7 
(d)     Undergraduate probability theory at the level of Hogg, Tanis & Zimmerman, Probability and Statistical Inference, 9th ed., Chapters 1-5 2. 

2.    Recommended 
(a)  Undergraduate physics at the level of Young and Freedman, University Physics, 14th ed., Basic mechanics – Chapters 1-16; Basic electricity and magnetism – Chapters 21-34 or Halliday, Resnick and Walker, Fundamentals of Physics, Basic mechanics – Chapters 1-16; basic E & M – Chapters 23-38 
(b)  Undergraduate mathematical biology at the level of Kot, Elements of mathematical ecology, 2001, Sections A, C, and E 
(c)  Undergraduate applied/engineering PDE at the level of Haberman, Applied Partial Differential Equations, 5th ed., especially Chapters 1-9 or Olver, Introduction to Partial Differential Equations, Chapters 1-7 
(d)  Undergraduate numerical methods at the level of Kincaid & Cheney, Numerical Analysis: Mathematics of Scientific Computing, 3rd ed., Chapters 1,3,4,6-9; some experience coding, e.g., using MATLAB, built-in ode/pde solvers, etc. 
(e)  Undergraduate nonlinear dynamics at the level of Strogatz, Nonlinear Dynamics and Chaos, 2nd ed., especially Chapters 1-3, 5-7, 9-11 
(f)  Undergraduate complex analysis at the level of Churchill and Brown, Complex Variables and Applications, 8th ed., Chapters 1-10

3.    Nice to have / wish list 
(a)  Random walks and diffusion at the level of Lawler, Random Walk and the Heat Equation, Sections 1.1-1.3; 2.1-2.5 
(b)  Graduate functional analysis at the level of Kreyszig, Introductory Functional Analysis with Applications, Chapters 1-9 
(c)  Graduate partial differential equations at the level of Evans, Partial Differential Equations, Chapters 1-5 or Salsa, Partial Differential Equations in Action, Chapters 1-8 
(d)  Graduate real analysis at the level of Royden and Fitzpatrick, Real Analysis, 4th ed., Chapters 1-8 
(e)  Graduate linear algebra at the level of Strang, Linear Algebra and its Applications, 4th ed., Chapters 1-7 
(f)  Graduate complex analysis at the level of Stein and Shakarchi, Complex Analysis; Chapters 1-5, 8

Application Procedure

SLMath is only able to support a limited number of students to attend this school.  Therefore, it is likely that only one student per institution will be funded by SLMath.

For eligibility and how to apply, see the Summer Graduate Schools homepage.

Venue

The location of the summer school is the University of Alaska in Fairbanks.