Program
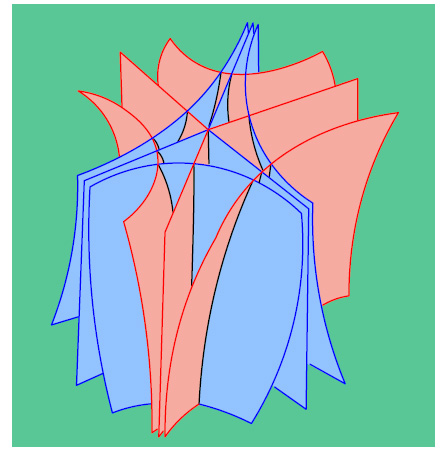
The stable and unstable foliations near a singular orbit of a pseudo- Anosov flow in 3 dimensions. Courtesy Michael Landry.
Low dimensional topology is a meeting place for ideas, objects and techniques that interact richly with each other, and generate implications for many parts of mathematics. Geometric structures, such as hyperbolic structures on 2- and 3-manifolds, interact with dynamical properties of flows and with analysis on parameter spaces such as the Teichmüller space of a surface or a foliation by surfaces. Combinatorial objects such as complexes of curves and their generalizations give us insight into the behavior of mapping class groups, which encapsulate the topological symmetries of a surface, as well as homeomorphism and diffeomorphism groups which blend topology and dynamics.
Seminal work of Thurston in the 1970’s brought many of these ideas together in new ways, and inspired multiple lines of work in the time since then, exploring different aspects of the relationship between geometry, topology, analysis and dynamics. Recent progress in these fields has taken each in new directions, suggesting that refocusing on their interactions will yield dividends towards progress on key problems within this central area and grow outwards towards its many connections with other areas of mathematics.
As examples of structural questions in the overlap of these areas: How do we classify Anosov and pseudo-Anosov flows on 3-manifolds up to orbit equivalence? Can we relate the dynamics of pseudo-Anosov flows on hyperbolic 3-manifolds to the geometry of the underlying 3-manifolds? Can we relate the leafwise Teichmuller theory of a foliation to geometric structures on the underlying 3-manifold? How well can we understand the subgroup structure of homeomorphism and diffeomorphism groups of surfaces? Can mapping class groups of infinite-type surfaces be harnessed to study dynamical questions?
The program will bring together experts in all these fields and younger researchers, who together can address these sorts of questions and open new areas for exploration.
Download a PDF flyer to share with your networks
Keywords and Mathematics Subject Classification (MSC)
Tags/Keywords
Surfaces
3-Manifolds
foliations
flows
homeomorphism groups
diffeomorphism groups
mapping class groups
Teichm¨uller theory
curve complexes
hyperbolic geometry
pseudo- Anosov
relative hyperbolicity
Minimal surface
pleated surface.
