Summer Graduate School

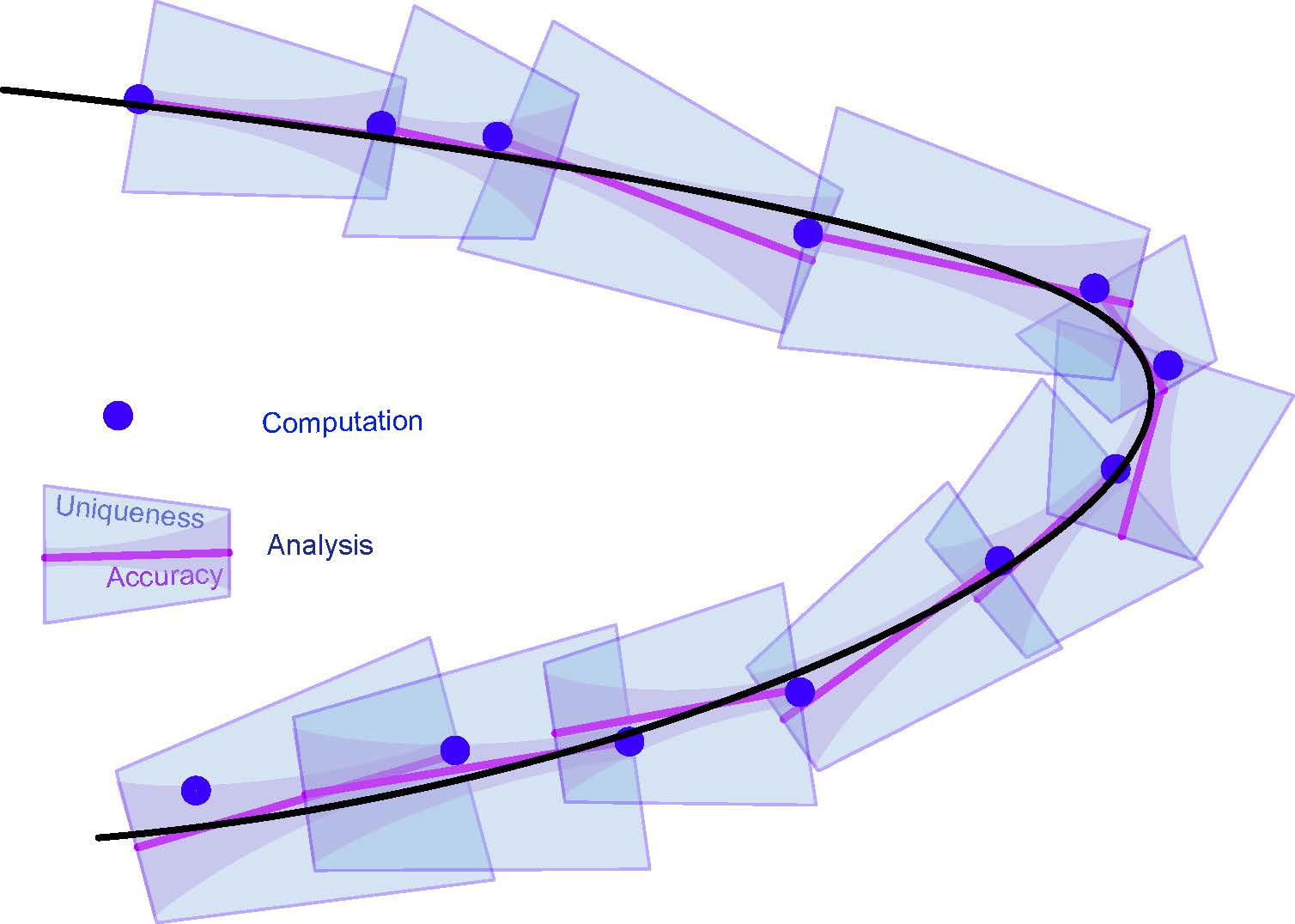
One of the core elements of applied mathematics is mathematical modeling, consisting of nonlinear equations, such as maps, and ordinary differential equations, and partial differential equations. Such models are widely used to describe complex phenomena in biology, physics, chemistry, meteorology, epidemiology, medicine, and many other fields. A fundamental difficulty arising in studying nonlinear models is that most cannot be solved in closed form. 

Computer assisted proofs are at the forefront of modern mathematics, and have led to many important recent mathematical advances. They provide a way of melding analytical techniques with numerical methods, in order to provide rigorous statements for mathematical models that could not be treated by either method alone. 

In this summer school, students will review standard computational and analytical techniques, learn to combine these techniques with more specialized methods of interval arithmetic and automatic differentiation, and apply these methods to establish rigorous results in otherwise intractable problems. They will start by learning to find rigorous bounds for intractable integrals and series solutions, establish existence and behavior of equilibrium solutions and periodic orbits for iterated maps, ordinary and partial differential equations, and to find accurate validated bounded for solutions of initial and boundary value problems. The course will be highly interactive, with students working through and presenting a series of exercises, culminating with a more substantial final project.

School Structure

There will be two lectures and two problem sessions each day. Students will work in groups of 3-4 on problem sessions.  In the second week, each group will prepare a week long project, to be presented on the last day of the program.

Prerequisites

Required: One semester of the following topics or practical equivalent:

• Introduction to programming: Students should have familiarity with basic programming, either through a course or equivalent experience.  We will be using Matlab and/or Julia, but we do not require students to have used it before.
• Undergraduate numerical analysis. Here is a  list of topics which can found in many books including Numerical Analysis by Tim Sauer (Pearson)
  • Floating point: representation of numbers and error
  • Solving equations: Bisection and Newton’s method; convergence and accuracy
  • Systems of equations: Gaussian elimination, LU factorization methods, condition number
  • Interpolation of data: Lagrange interpolation, Chebyshev interpolation, error estimates
  • Least squares methods
• Undergraduate real analysis

Recommended: Basic theory of Banach spaces, and familiarity with the Contraction Mapping Theorem, as found in a standard graduate analysis or functional analysis book. For example, see Introductory functional analysis with applications by Erwin Kreyszig.

• Ch. 1 Metric spaces
• Ch. 2 Normed spaces, Banach spaces
• Ch. 5 Banach fixed point theorem

Application Procedure

For eligibility and how to apply, see the Summer Graduate Schools homepage.