MSRI-UP 2020: Branched Covers of Curves
| Home | Research Topic | People | Colloquia | Research Projects | Pictures |
The theme of the 2020 MSRI-UP was “Branched Covers of Curves" and the research leader was Dr. Edray Goins, Professor of Mathematics at Pomona College. The research program focused on Galois Theory of curves, i.e. the realization of certain finite groups as the symmetries of maps from one curve to another. Students worked on a variety of problems ranging from the explicit construction of covers for a given group to visualizing such covers as exotic surfaces which are self-intersections of the sphere and the torus. The research groups focused on Belyi maps, Dessin d’Enfants, Origami, and Shabat polynomials; while working in a variety of areas such as Galois theory, monodromy groups, number theory, and Riemann surfaces.
Download all abstracts (PDF) / Download all abstracts (TeX file, via zip)
Download final presentation day program (PDF)
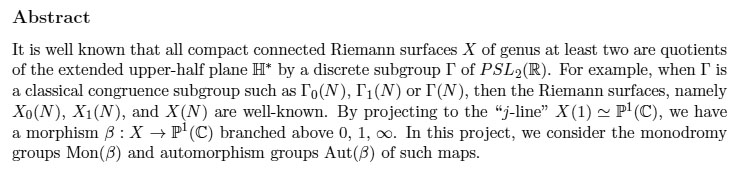
Project #1: Automorphism Groups and Monodromy of Classical Modular Curves
Group Members: Samuel Heard, Fabian Ramirez, and Vanessa Sun
Project #2: Explicit Constructions of Finite Groups as Monodromy Groups
Group Members: Ra-Zakee Muhammad, Javier Santiago, and Eyob Tsegaye

Project #3: Dessin d'Enfants from Cartographic Groups
Group Members: Nicholas Arosemena, Yaren Euceda, and Ashly Powell

Project #4: Visualizing Toroidal Belyĭ Pairs
Group Members: Deion Elzie, Mikaela Nishida, and Cameron Thomas

Project #5: Computing Monodromy of Toroidal Belyĭ Pairs
Group Members: Rebecca Lopez and Chidera Okenwa

Project #6: To and From 2-Generated Groups and Origamis: Starting from Square One
Group Members: Sarai Gonzalez, Elisa Rodriguez, and William Sablan

