-
Program Probability and Statistics of Discrete Structures
Organizers: Louigi Addario-Berry (McGill University), Christina Goldschmidt (University of Oxford), Po-Ling Loh (University of Cambridge), Gábor Lugosi (Pompeu Fabra University), Dana Randall (Georgia Institute of Technology), LEAD Remco van der Hofstad (Technische Universiteit Eindhoven)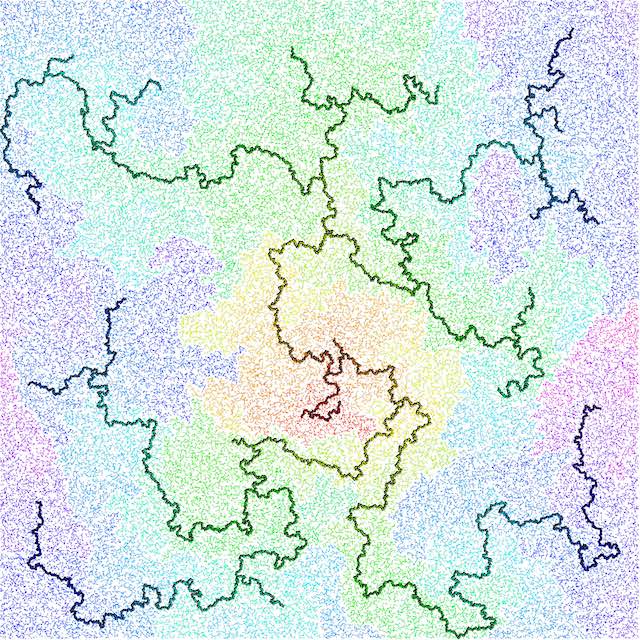 The minimum spanning tree of 100,000 uniformly random points. Colors encode graph distance from the root, which is red. Black points are those whose removal would disconnect at least 5% of the points from the rest.
The minimum spanning tree of 100,000 uniformly random points. Colors encode graph distance from the root, which is red. Black points are those whose removal would disconnect at least 5% of the points from the rest.This program is devoted to the study of the probabilistic and statistical properties of such networks. Central tools include graphon theory for dense graphs, local weak convergence for sparse graphs, and scaling limits for the critical behavior of graphs or stochastic processes on them. The program is aimed at pure and applied mathematicians interested in network problems.
Updated on Dec 19, 2024 09:02 AM PST -
Program Extremal Combinatorics
Organizers: LEAD David Conlon (California Institute of Technology), LEAD Jacob Fox (Stanford University), Penny Haxell (University of Waterloo), János Pach (Alfréd Rényi Institute of Mathematics), Maya Stein (Universidad de Chile), Andrew Suk (University of California, San Diego)
Extremal combinatorics concerns itself with problems about how large or small a finite collection of objects can be while satisfying certain conditions. Questions of this type arise naturally across mathematics, so this area has close connections and interactions with a broad array of other fields, including number theory, group theory, model theory, probability, statistical physics, optimization, and theoretical computer science.
Updated on Dec 16, 2024 03:09 PM PST -
Program New Frontiers in Curvature: Flows, General Relativity, Minimal Submanifolds, and Symmetry
Organizers: LEAD Ailana Fraser (University of British Columbia), Lan-Hsuan Huang (University of Connecticut), Richard Schoen (University of California, Irvine), LEAD Catherine Searle (Wichita State University), Lu Wang (Yale University), Guofang Wei (University of California, Santa Barbara)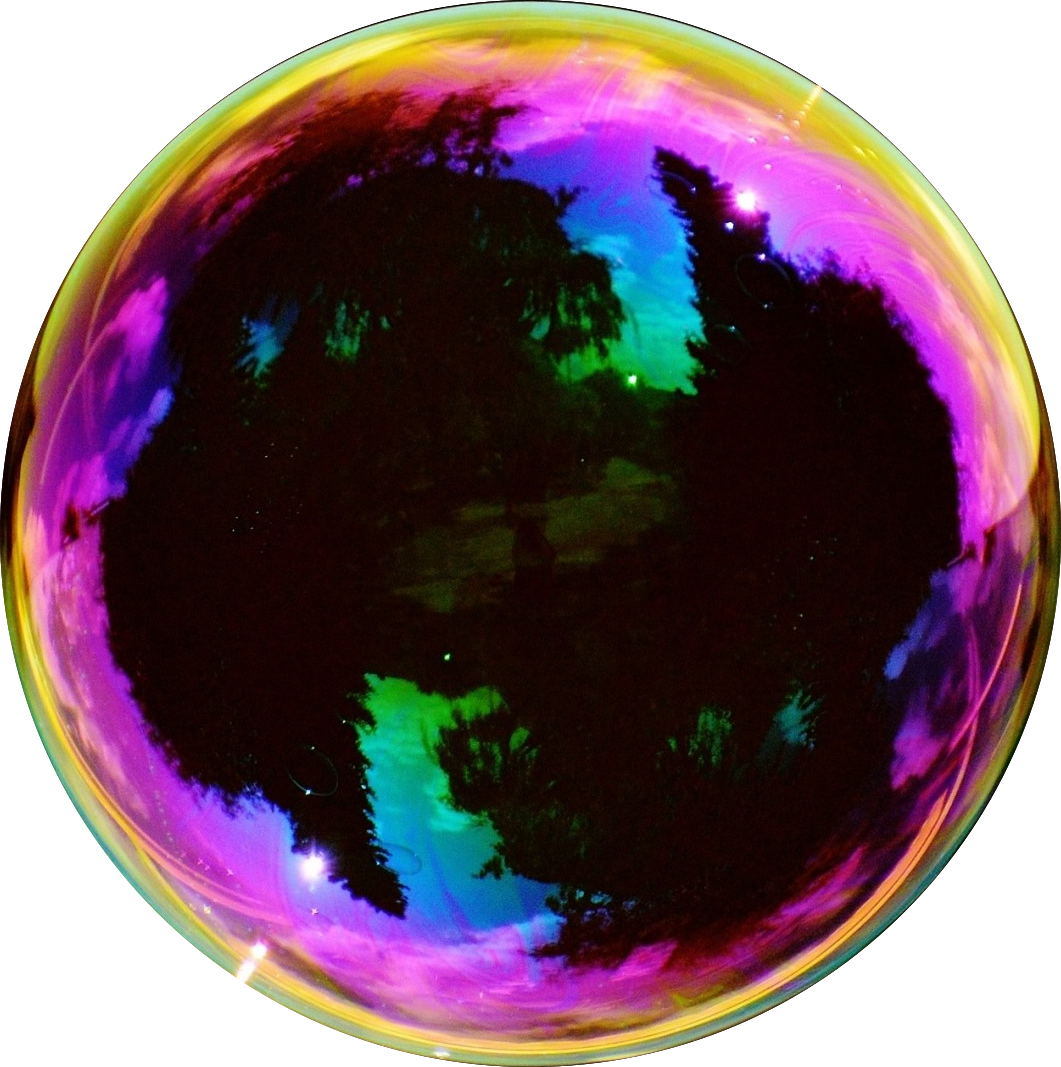 Soap bubble: equilibrium solution of the mean curvature flow and constant curvature surface.
Soap bubble: equilibrium solution of the mean curvature flow and constant curvature surface.Geometry, PDE, and Relativity are subjects that have shown intriguing interactions in the past several decades, while simultaneously diverging, each with an ever growing number of branches. Recently, several major breakthroughs have been made in each of these fields using techniques and ideas from the others.
This program is aimed at connecting various branches of Geometry, PDE, and Relativity and at enhancing collaborations across these disciplines and will include four main topics: Geometric Flows, Geometric problems in Mathematical Relativity, Global Riemannian Geometry, and Minimal Submanifolds. Specifically the program focuses on a central goal, which is to advance our knowledge toward Riemannian (sub)manifolds under geometric conditions, such as curvature lower bounds, by developing techniques in, for example, geometric flows and minimal submanifolds and further fostering new connections.
Updated on Sep 19, 2024 09:19 AM PDT -
Program Special Geometric Structures and Analysis
Organizers: Eleonora Di Nezza (Institut de Mathématiques de Jussieu; École Normale Supérieure), LEAD Mark Haskins (Duke University), Tristan Riviere (ETH Zurich), Song Sun (Zhejiang University; University of California, Berkeley), Xuwen Zhu (Northeastern University)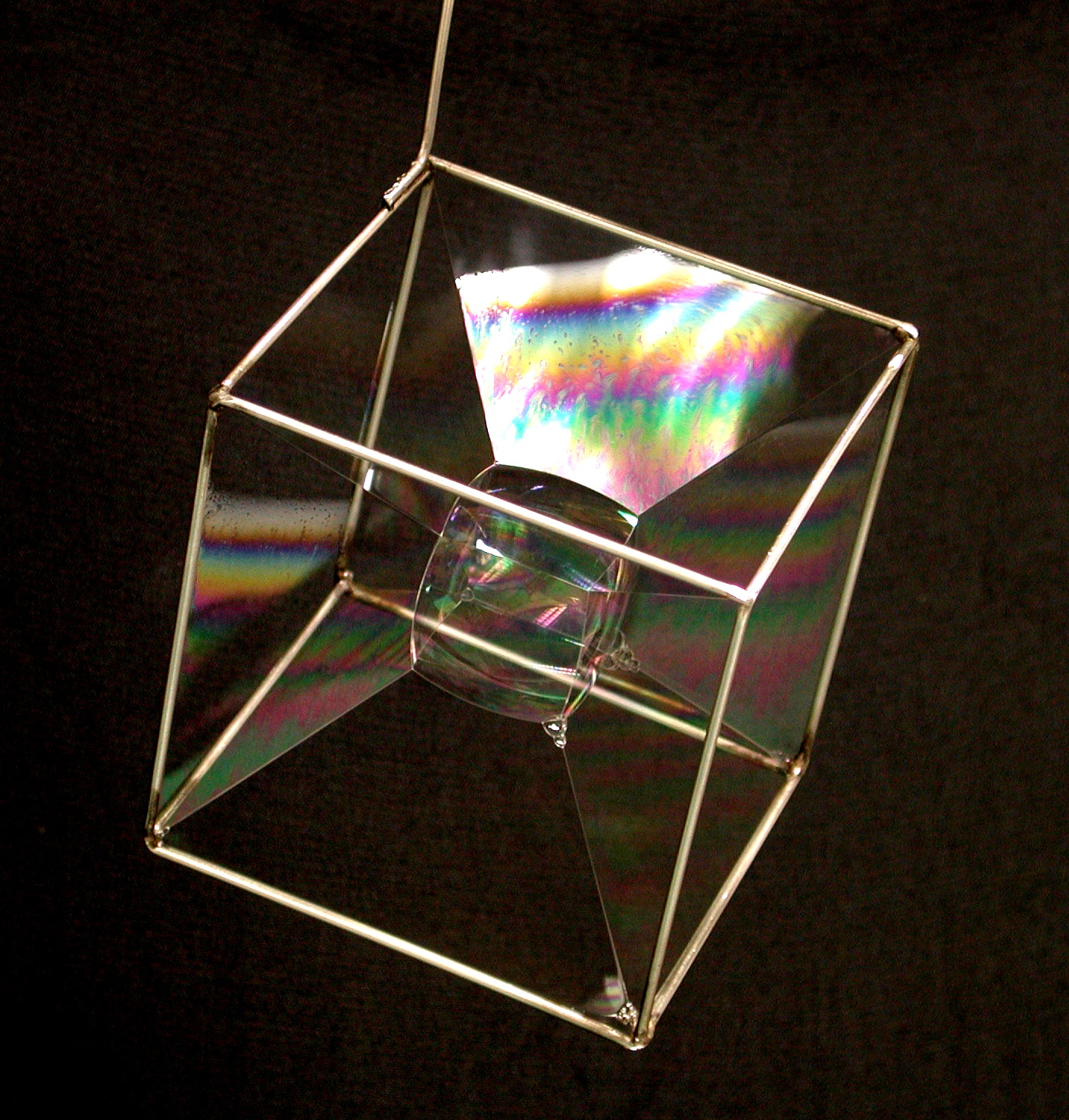 “Plateau’s Memory ” (by A. van der Net): A soap film with singularities
“Plateau’s Memory ” (by A. van der Net): A soap film with singularitiesThis program sits at the intersection between differential geometry and analysis but also connects to several other adjacent mathematical fields and to theoretical physics. Differential geometry aims to answer questions about very regular geometric objects (smooth Riemannian manifolds) using the tools of differential calculus. A fundamental object is the curvature tensor of a Riemannian metric: an algebraically complicated object that involves 2nd partial derivatives of the metric. Many questions in differential geometry can therefore be translated into questions about the existence or properties of the solutions of systems of (often) nonlinear partial differential equations (PDEs). The PDE systems that arise in geometry have historically stimulated the development of powerful new analytic methods. In most cases the nonlinearity of these systems makes ‘closed form’ expressions for a solution impossible: instead more abstract methods must be employed.
Updated on Apr 10, 2025 01:21 PM PDT -
Program Quantum Symmetries Reunion
 The study of tensor categories involves the interplay of representation theory, combinatorics, number theory, and low dimensional topology (from a string diagram calculation, describing the 3-dimensional bordism 2-category [arXiv:1411.0945]).
The study of tensor categories involves the interplay of representation theory, combinatorics, number theory, and low dimensional topology (from a string diagram calculation, describing the 3-dimensional bordism 2-category [arXiv:1411.0945]).Symmetry, as formalized by group theory, is ubiquitous across mathematics and science. Classical examples include point groups in crystallography, Noether's theorem relating differentiable symmetries and conserved quantities, and the classification of fundamental particles according to irreducible representations of the Poincaré group and the internal symmetry groups of the standard model. However, in some quantum settings, the notion of a group is no longer enough to capture all symmetries. Important motivating examples include Galois-like symmetries of von Neumann algebras, anyonic particles in condensed matter physics, and deformations of universal enveloping algebras. The language of tensor categories provides a unified framework to discuss these notions of quantum symmetry.
Updated on Jun 27, 2024 12:12 PM PDT -
Program Complementary Program 2023-24
The Complementary Program has a limited number of memberships that are open to mathematicians whose interests are not closely related to the core programs; special consideration is given to mathematicians who are partners of an invited member of a core program.
Updated on Sep 26, 2023 11:36 AM PDT -
Program Commutative Algebra
Organizers: Aldo Conca (Università di Genova), Steven Cutkosky (University of Missouri), LEAD Claudia Polini (University of Notre Dame), Claudiu Raicu (University of Notre Dame), Steven Sam (University of California, San Diego), Kevin Tucker (University of Illinois at Chicago), Claire Voisin (Collège de France; Institut de Mathématiques de Jussieu)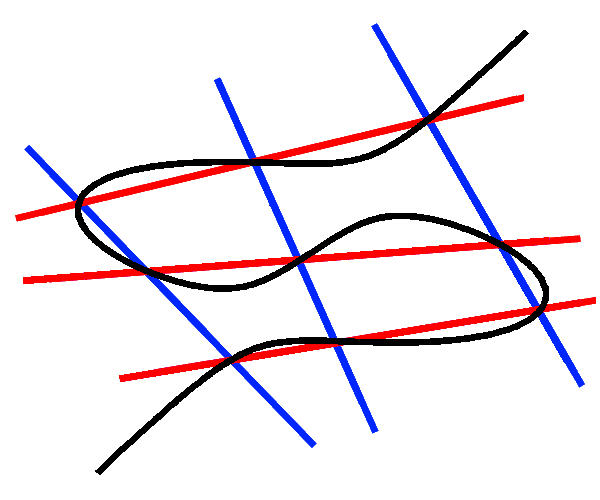 Image for theorem about 9 point on cubic curve, the special case of Cayley–Bacharach theorem.
Image for theorem about 9 point on cubic curve, the special case of Cayley–Bacharach theorem.Commutative algebra is, in its essence, the study of algebraic objects, such as rings and modules over them, arising from polynomials and integral numbers. It has numerous connections to other fields of mathematics including algebraic geometry, algebraic number theory, algebraic topology and algebraic combinatorics. Commutative Algebra has witnessed a number of spectacular developments in recent years, including the resolution of long-standing problems, with new techniques and perspectives leading to an extraordinary transformation in the field. The main focus of the program will be on these developments. These include the recent solution of Hochster's direct summand conjecture in mixed characteristic that employs the theory of perfectoid spaces, a new approach to the Buchsbaum--Eisenbud--Horrocks conjecture on the Betti numbers of modules of finite length, recent progress on the study of Castelnuovo--Mumford regularity, the proof of Stillman's conjecture and ongoing work on its effectiveness, a novel strategy to Green's conjecture on the syzygies of canonical curves based on the study of Koszul modules and their generalizations, new developments in the study of various types of multiplicities, theoretical and computational aspects of Gröbner bases, and the implicitization problem for Rees algebras and its applications.
Updated on Jan 02, 2024 10:36 AM PST -
Program Noncommutative Algebraic Geometry
Organizers: Wendy Lowen (Universiteit Antwerpen), Alex Perry (University of Michigan), LEAD Alexander Polishchuk (University of Oregon), Susan Sierra (University of Edinburgh), Michel VAN DEN BERGH (Hasselt University), Špela Špenko (Université Libre de Bruxelles) Optical illusion staircase
Optical illusion staircaseDerived categories of coherent sheaves on algebraic varieties were originally conceived as technical tools for studying cohomology, but have since become central objects in fields ranging from algebraic geometry to mathematical physics, symplectic geometry, and representation theory. Noncommutative algebraic geometry is based on the idea that any category sufficiently similar to the derived category of a variety should be regarded as (the derived category of) a “noncommutative algebraic variety”; examples include semiorthogonal components of derived categories, categories of matrix factorizations, and derived categories of noncommutative dg-algebras. This perspective has led to progress on old problems, as well as surprising connections between seemingly unrelated areas. In recent years there have been great advances in this domain, including new tools for constructing semiorthogonal decompositions and derived equivalences, progress on conjectures relating birational geometry and singularities to derived categories, constructions of moduli spaces from noncommutative varieties, and instances of homological mirror symmetry for noncommutative varieties. The goal of this program is to explore and expand upon these developments.
Updated on Jan 22, 2024 12:22 PM PST -
Program Mathematics and Computer Science of Market and Mechanism Design
Organizers: Martin Bichler (TU München), Péter Biró (KRTK – Institute of Economics), Michal Feldman (Tel-Aviv University), Nicole Immorlica (Microsoft Research), LEAD Scott Kominers (Harvard Business School), Shengwu Li (Harvard University), Paul Milgrom (Stanford University), Alvin Roth (Stanford University), Eva Tardos (Cornell University)
In recent years, economists and computer scientists have collaborated with mathematicians, operations research experts, and practitioners to improve the design and operations of real-world marketplaces. Such work relies on robust feedback between theory and practice, inspiring new mathematics closely linked – and directly applicable – to market and mechanism design questions. This cross-disciplinary program seeks to expand the domains in which existing market design solutions can be applied; address foundational questions regarding our ways of developing and evaluating mechanisms; and build useful analytic frameworks for applying theory to practical marketplace design.
Updated on Oct 18, 2023 09:04 AM PDT -
Program Algorithms, Fairness, and Equity
Organizers: Vincent Conitzer (Carnegie Mellon University), Moon Duchin (Tufts University), Bettina Klaus (University of Lausanne), Jonathan Mattingly (Duke University), LEAD Wesley Pegden (Carnegie Mellon University)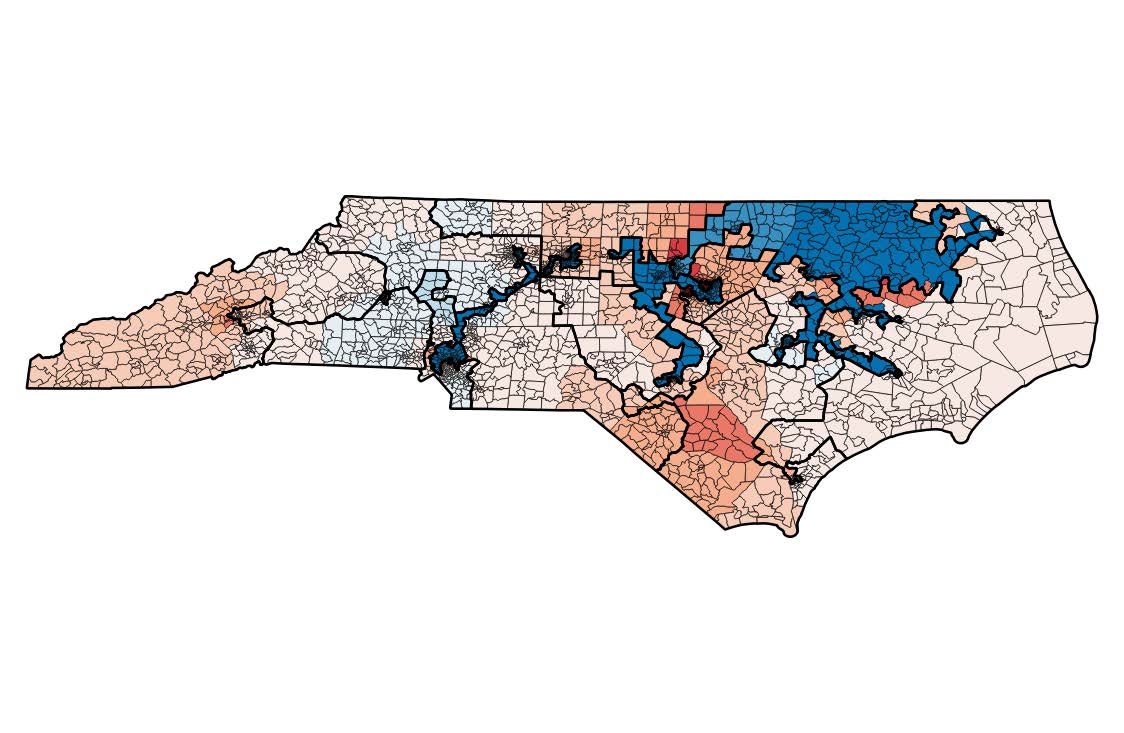 <p>A graphical representation of a Markov Chain fairness analysis of a political districting in North Carolina from Chin, Herschlag, Mattingly</p>
<p>A graphical representation of a Markov Chain fairness analysis of a political districting in North Carolina from Chin, Herschlag, Mattingly</p>This program aims to bring together researchers working at the interface of fairness and computation. This interface has been the site of intensive research effort in mechanism design, in research on partitioning problems related to political districting problems, and in research on ways to address issues of fairness and equity in the context of machine learning algorithms.
These areas each approach the relationship between mathematics and fairness from a distinct perspective. In mechanism design, algorithms are a tool to achieve outcomes with mathematical guarantees of various notions of fairness. In machine learning, we perceive failures of fairness as an undesirable side effect of learning approaches, and seek mathematical approaches to understand and mitigate these failures. And in partitioning problems like political districting, we often seek mathematical tools to evaluate the fairness of human decisions.
This program will explore progress in these areas while also providing a venue for overlapping perspectives. The topics workshop “Randomization, neutrality, and fairness” will explore the common role randomness and probability has played in these lines of work.
Updated on Aug 24, 2023 07:26 AM PDT -
Program Complementary Program 2022-23
Updated on Aug 07, 2023 05:47 PM PDT -
Program Mathematical Problems in Fluid Dynamics, part 2
Organizers: Thomas Alazard (Ecole Normale Supérieure Paris-Saclay; Centre National de la Recherche Scientifique (CNRS)), Hajer Bahouri (Laboratoire Jacques-Louis Lions; Centre National de la Recherche Scientifique (CNRS)), Mihaela Ifrim (University of Wisconsin-Madison), Igor Kukavica (University of Southern California), David Lannes (Institut de Mathématiques de Bordeaux; Centre National de la Recherche Scientifique (CNRS)), Daniel Tataru (University of California, Berkeley)PROGRAM DESCRIPTION
Fluid dynamics is one of the classical areas of partial differential equations, and has been the subject of extensive research over hundreds of years. It is perhaps one of the most challenging and exciting fields of scientific pursuit simply because of the complexity of the subject and the endless breadth of applications.
The focus of the program is on incompressible fluids, where water is a primary example. The fundamental equations in this area are the well-known Euler equations for inviscid fluids, and the Navier-Stokes equations for the viscous fluids. Relating the two is the problem of the zero viscosity limit, and its connection to the phenomena of turbulence. Water waves, or more generally interface problems in fluids, represent another target area for the program. Both theoretical and numerical aspects will be considered.
Updated on Oct 20, 2023 09:39 AM PDT -
Program Simons Bridge Postdoctoral Fellowship 2022/23
Updated on Feb 10, 2022 10:34 AM PST -
Program Algebraic Cycles, L-Values, and Euler Systems
Organizers: Henri Darmon (McGill University), Ellen Eischen (University of Oregon), LEAD Benjamin Howard (Boston College), David Loeffler (UniDistance Suisse), Christopher Skinner (Princeton University), Sarah Zerbes (ETH Zürich), Wei Zhang (Massachusetts Institute of Technology)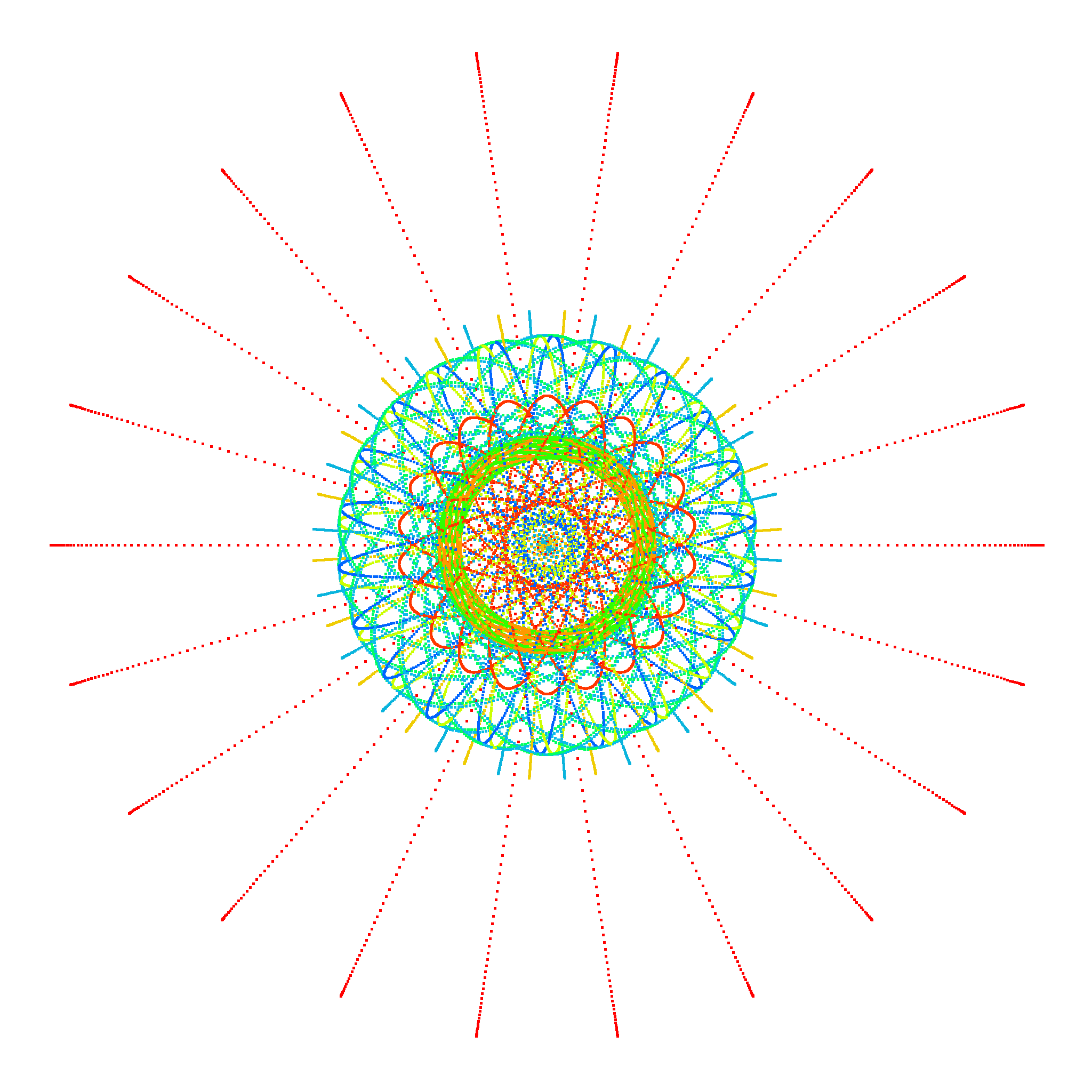 Some Gaussian periods for the 255,255-th cyclotomic extension. Image credit: E. Eischen, based on earlier work by W. Duke, S. R. Garcia, T. Hyde, and R. Lutz
Some Gaussian periods for the 255,255-th cyclotomic extension. Image credit: E. Eischen, based on earlier work by W. Duke, S. R. Garcia, T. Hyde, and R. LutzThe fundamental conjecture of Birch and Swinnerton-Dyer relating the Mordell–Weil ranks of elliptic curves to their L-functions is one of the most important and motivating problems in number theory. It resides at the heart of a collection of important conjectures (due especially to Deligne, Beilinson, Bloch and Kato) that connect values of L-functions and their leading terms to cycles and Galois cohomology groups.
The study of special algebraic cycles on Shimura varieties has led to progress in our understanding of these conjectures. The arithmetic intersection numbers and the p-adic regulators of special cycles are directly related to the values and derivatives of L-functions, as shown in the pioneering theorem of Gross-Zagier and its p-adic avatars for Heegner points on modular curves. The cohomology classes of special cycles (and related constructions such as Eisenstein classes) form the foundation of the theory of Euler systems, providing one of the most powerful methods known to prove vanishing or finiteness results for Selmer groups of Galois representations.
The goal of this semester is to bring together researchers working on different aspects of this young but fast-developing subject, and to make progress on understanding the mysterious relations between L-functions, Euler systems, and algebraic cycles.
Updated on Oct 20, 2023 10:26 AM PDT -
Program Diophantine Geometry
Organizers: Jennifer Balakrishnan (Boston University), Mirela Ciperiani (University of Texas, Austin), Philipp Habegger (University of Basel), Wei Ho (Institute for Advanced Study), LEAD Hector Pasten (Pontificia Universidad Católica de Chile), Yunqing Tang (University of California, Berkeley), Shou-Wu Zhang (Princeton University)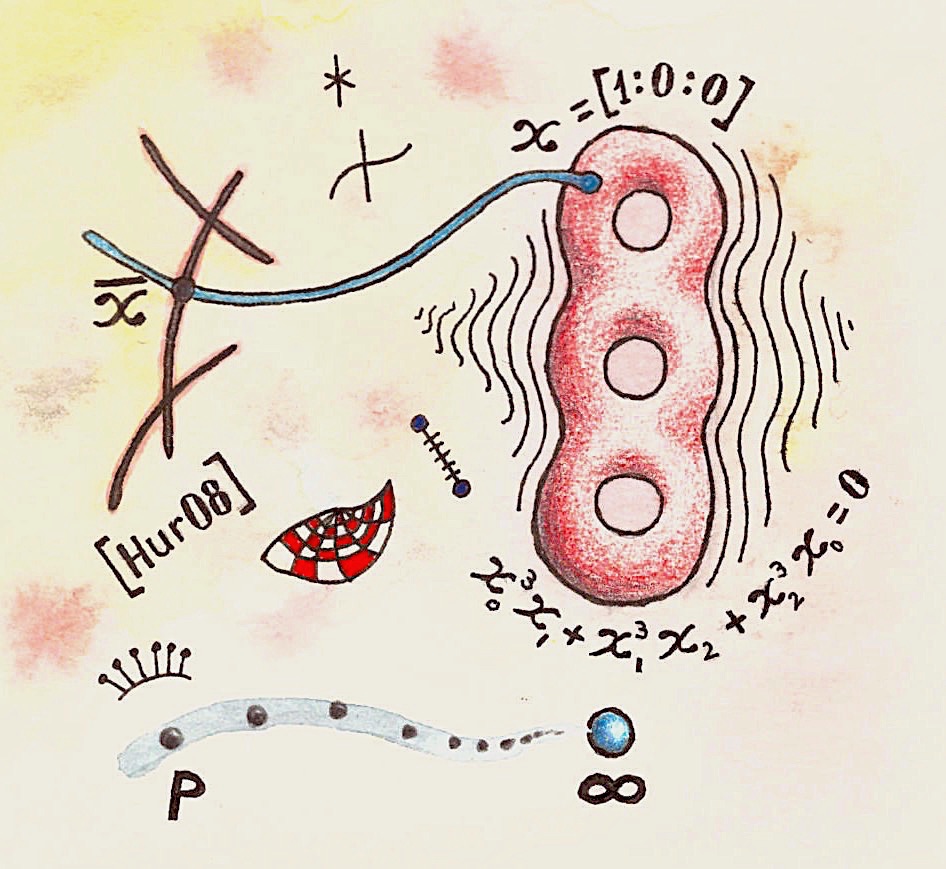 A rational point on a curve of genus 3
A rational point on a curve of genus 3While the study of rational solutions of diophantine equations initiated thousands of years ago, our knowledge on this subject has dramatically improved in recent years. Especially, we have witnessed spectacular progress in aspects such as height formulas and height bounds for algebraic points, automorphic methods, unlikely intersection problems, and non-abelian and p-adic approaches to algebraic degeneracy of rational points. All these groundbreaking advances in the study of rational and algebraic points in varieties will be the central theme of the semester program “Diophantine Geometry” at MSRI. The main purpose of this program is to bring together experts as well as enthusiastic young researchers to learn from each other, to initiate and continue collaborations, to update on recent breakthroughs, and to further advance the field by making progress on fundamental open problems and by developing further connections with other branches of mathematics. We trust that younger mathematicians will greatly contribute to the success of the program with their new ideas. It is our hope that this program will provide a unique opportunity for women and underrepresented groups to make outstanding contributions to the field, and we strongly encourage their participation.
Updated on Oct 20, 2023 10:29 AM PDT -
Program Floer Homotopy Theory
Organizers: Mohammed Abouzaid (Columbia University), Andrew Blumberg (Columbia University), Kristen Hendricks (Rutgers University), Robert Lipshitz (University of Oregon), LEAD Ciprian Manolescu (Stanford University), Nathalie Wahl (University of Copenhagen) Illustrated by Nathalie Wahl
Illustrated by Nathalie WahlThe development of Floer theory in its early years can be seen as a parallel to the emergence of algebraic topology in the first half of the 20th century, going from counting invariants to homology groups, and beyond that to the construction of algebraic structures on these homology groups and their underlying chain complexes. In continuing work that started in the latter part of the 20th century, algebraic topologists and homotopy theorists have developed deep methods for refining these constructions, motivated in large part by the application of understanding the classification of manifolds. The goal of this program is to relate these developments to Floer theory with the dual aims of (i) making progress in understanding symplectic and low-dimensional topology, and (ii) providing a new set of geometrically motivated questions in homotopy theory.
Updated on Oct 20, 2023 10:47 AM PDT -
Program Analytic and Geometric Aspects of Gauge Theory
Organizers: Laura Fredrickson (University of Oregon), Rafe Mazzeo (Stanford University), Tomasz Mrowka (Massachusetts Institute of Technology), Laura Schaposnik (University of Illinois at Chicago), LEAD Thomas Walpuski (Humboldt-Universität)
The mathematics and physics around gauge theory have, since their first interaction in the mid 1970’s, prompted tremendous developments in both mathematics and physics. Deep and fundamental tools in partial differential equations have been developed to provide rigorous foundations for the mathematical study of gauge theories. This led to ongoing revolutions in the understanding of manifolds of dimensions 3 and 4 and presaged the development of symplectic topology. Ideas from quantum field theory have provided deep insights into new directions and conjectures on the structure of gauge theories and suggested many potential applications. The focus of this program will be those parts of gauge theory which hold promise for new applications to geometry and topology and require development of new analytic tools for their study.
Updated on Oct 20, 2023 10:50 AM PDT -
Program Definability, Decidability, and Computability in Number Theory, part 2
Organizers: Valentina Harizanov (George Washington University), Barry Mazur (Harvard University), Russell Miller (Queens College, CUNY; CUNY, Graduate Center), Jonathan Pila (University of Oxford), Thomas Scanlon (University of California, Berkeley), Alexandra Shlapentokh (East Carolina University) Title page of Diophantus' Arithmetica - ETH Zurich
Title page of Diophantus' Arithmetica - ETH ZurichThis program is focused on the two-way interaction of logical ideas and techniques, such as definability from model theory and decidability from computability theory, with fundamental problems in number theory. These include analogues of Hilbert's tenth problem, isolating properties of fields of algebraic numbers which relate to undecidability, decision problems around linear recurrence and algebraic differential equations, the relation of transcendence results and conjectures to decidability and decision problems, and some problems in anabelian geometry and field arithmetic. We are interested in this specific interface across a range of problems and so intend to build a semester which is both more topically focused and more mathematically broad than a typical MSRI program.
Updated on Dec 21, 2021 09:51 AM PST -
Program Complementary Program 2021-22
The Complementary Program has a limited number of memberships that are open to mathematicians whose interests are not closely related to the core programs; special consideration is given to mathematicians who are partners of an invited member of a core program.
Updated on May 03, 2022 02:54 PM PDT -
Program Simons Bridge Postdoctoral Fellowship 2021/22
Updated on Feb 10, 2022 10:34 AM PST -
Program Higher Categories and Categorification, Part Two
Organizers: David Ayala (Montana State University), Clark Barwick (University of Edinburgh), David Nadler (University of California, Berkeley), LEAD Emily Riehl (Johns Hopkins University), Marcy Robertson (University of Melbourne), Peter Teichner (Max-Planck-Institut für Mathematik), Dominic Verity (Macquarie University) swallowtail identity
swallowtail identityThough many of the ideas in higher category theory find their origins in homotopy theory — for instance as expressed by Grothendieck’s “homotopy hypothesis” — the subject today interacts with a broad spectrum of areas of mathematical research. Unforeseen descent, or local-to-global formulas, for familiar objects can be articulated in terms of higher invertible morphisms. Compatible associative deformations of a sequence of maps of spaces, or derived schemes, can putatively be represented by higher categories, as Koszul duality for E_n-algebras suggests. Higher categories offer unforeseen characterizing universal properties for familiar constructions such as K-theory. Manifold theory is natively connected to higher category theory and adjunction data, a connection that is most famously articulated by the recently proven Cobordism Hypothesis.
In parallel, the idea of "categorification'' is playing an increasing role in algebraic geometry, representation theory, mathematical physics, and manifold theory, and higher categorical structures also appear in the very foundations of mathematics in the form of univalent foundations and homotopy type theory. A central mission of this semester will be to mitigate the exorbitantly high "cost of admission'' for mathematicians in other areas of research who aim to apply higher categorical technology and to create opportunities for potent collaborations between mathematicians from these different fields and experts from within higher category theory.Updated on Jun 06, 2022 12:39 PM PDT -
Program The Analysis and Geometry of Random Spaces
Organizers: LEAD Mario Bonk (University of California, Los Angeles), Joan Lind (University of Tennessee), Steffen Rohde (University of Washington), Eero Saksman (University of Helsinki), Fredrik Viklund (Royal Institute of Technology), Jang-Mei Wu (University of Illinois at Urbana-Champaign)
This program is devoted to the investigation of universal analytic and geometric objects that arise from natural probabilistic constructions, often motivated by models in mathematical physics. Prominent examples for recent developments are the Schramm-Loewner evolution, the continuum random tree, Bernoulli percolation on the integers, random surfaces produced by Liouville Quantum Gravity, and Jordan curves and dendrites obtained from random conformal weldings and laminations. The lack of regularity of these random structures often results in a failure of classical methods of analysis. One goal of this program is to enrich the analytic toolbox to better handle these rough structures.
Updated on Dec 21, 2021 12:37 PM PST -
Program Complex Dynamics: from special families to natural generalizations in one and several variables
Organizers: LEAD Sarah Koch (University of Michigan), Jasmin Raissy (Institut de Mathématiques de Bordeaux), Dierk Schleicher (Université d'Aix-Marseille (AMU)), Mitsuhiro Shishikura (Kyoto University), Dylan Thurston (Indiana University) The mating of these two dendritic Julia sets is equal to the Julia set of a rational map of degree 2; that Julia set is equal to the entire Riemann sphere. Picture by Arnaud Chéritat
The mating of these two dendritic Julia sets is equal to the Julia set of a rational map of degree 2; that Julia set is equal to the entire Riemann sphere. Picture by Arnaud ChéritatHolomorphic dynamics is a vibrant field of mathematics that has seen profound progress over the past 40 years. It has numerous interconnections to other fields of mathematics and beyond.
Our semester will focus on three selected classes of dynamical systems: rational maps (postcritically finite and beyond); transcendental maps; and maps in several complex variables. We will put particular emphasis on the interactions between each these, and on connections with adjacent areas of mathematics.
Updated on Oct 20, 2023 01:06 PM PDT -
Program Universality and Integrability in Random Matrix Theory and Interacting Particle Systems
Organizers: LEAD Ivan Corwin (Columbia University), Percy Deift (New York University, Courant Institute), Ioana Dumitriu (University of California, San Diego), Alice Guionnet (École Normale Supérieure de Lyon), Alexander Its (Indiana University--Purdue University), Herbert Spohn (Technische Universität München), Horng-Tzer Yau (Harvard University)
The past decade has seen tremendous progress in understanding the behavior of large random matrices and interacting particle systems. Complementary methods have emerged to prove universality of these behaviors, as well as to probe their precise nature using integrable, or exactly solvable models. This program seeks to reinforce and expand the fruitful interaction at the interface of these areas, as well as to showcase some of the important developments and applications of the past decade.
Updated on Oct 20, 2023 01:11 PM PDT -
Program Complementary Program 2020-21
The Complementary Program has a limited number of memberships that are open to mathematicians whose interests are not closely related to the core programs; special consideration is given to mathematicians who are partners of an invited member of a core program.
Updated on Jul 14, 2021 09:02 AM PDT -
Program Mathematical problems in fluid dynamics
Organizers: Thomas Alazard (Ecole Normale Supérieure Paris-Saclay; Centre National de la Recherche Scientifique (CNRS)), Hajer Bahouri (Laboratoire Jacques-Louis Lions; Centre National de la Recherche Scientifique (CNRS)), Mihaela Ifrim (University of Wisconsin-Madison), Igor Kukavica (University of Southern California), David Lannes (Institut de Mathématiques de Bordeaux; Centre National de la Recherche Scientifique (CNRS)), LEAD Daniel Tataru (University of California, Berkeley)
All scientific activities in this program will be available online so that those who can't attend in person are able to participate. If you are not a member of the program and would like to participate in any of the online activities, please fill out this REGISTRATION FORM.
PROGRAM DESCRIPTION
Fluid dynamics is one of the classical areas of partial differential equations, and has been the subject of extensive research over hundreds of years. It is perhaps one of the most challenging and exciting fields of scientific pursuit simply because of the complexity of the subject and the endless breadth of applications.
The focus of the program is on incompressible fluids, where water is a primary example. The fundamental equations in this area are the well-known Euler equations for inviscid fluids, and the Navier-Stokes equations for the viscous fluids. Relating the two is the problem of the zero viscosity limit, and its connection to the phenomena of turbulence. Water waves, or more generally interface problems in fluids, represent another target area for the program. Both theoretical and numerical aspects will be considered.
Updated on Oct 20, 2023 03:21 PM PDT -
Program Random and Arithmetic Structures in Topology -- Virtual Semester
Organizers: Nicolas Bergeron (École Normale Supérieure), Jeffrey Brock (Yale University), Alexander Furman (University of Illinois at Chicago), Tsachik Gelander (Weizmann Institute of Science), Ursula Hamenstädt (Rheinische Friedrich-Wilhelms-Universität Bonn), Fanny Kassel (Institut des Hautes Études Scientifiques (IHES)), LEAD Alan Reid (Rice University)
Until further notice, the MSRI building will only be open to a small group of essential staff and members of the Fall 2020 scientific programs.
All scientific activities in this program will be available online so that those who can't attend in person are able to participate. If you are not a member of the program and would like to participate in any of the online activities, please fill out this REGISTRATION FORM.
Updated on Sep 21, 2020 04:57 PM PDT -
Program Decidability, definability and computability in number theory: Part 1 - Virtual Semester
Organizers: LEAD Valentina Harizanov (George Washington University), Maryanthe Malliaris (University of Chicago), Barry Mazur (Harvard University), Russell Miller (Queens College, CUNY; CUNY, Graduate Center), Jonathan Pila (University of Oxford), Thomas Scanlon (University of California, Berkeley), LEAD Alexandra Shlapentokh (East Carolina University), Carlos Videla (Mount Royal University) Title page of Diophantus' Arithmetica - ETH Zurich
Title page of Diophantus' Arithmetica - ETH ZurichUntil further notice, the MSRI building will only be open to a small group of essential staff and members of the Fall 2020 scientific programs.
All scientific activities in this program will be available online so that those who can't attend in person are able to participate. If you are not a member of the program and would like to participate in any of the online activities, please fill out this REGISTRATION FORM.
Updated on Oct 29, 2020 10:47 AM PDT -
Program Quantum Symmetries
Organizers: Vaughan Jones (Vanderbilt University), LEAD Scott Morrison (Australian National University), Victor Ostrik (University of Oregon), Emily Peters (Loyola University), Eric Rowell (Texas A & M University), LEAD Noah Snyder (Indiana University), Chelsea Walton (Rice University)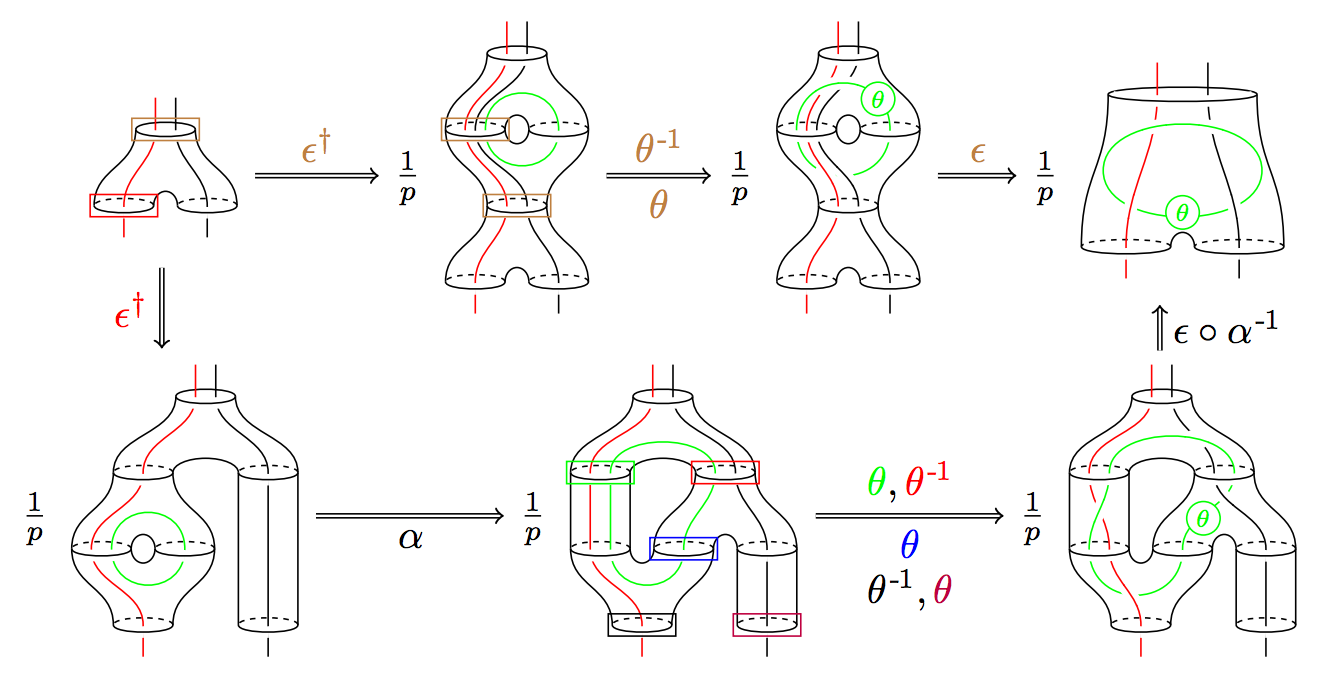 The study of tensor categories involves the interplay of representation theory, combinatorics, number theory, and low dimensional topology (from a string diagram calculation, describing the 3-dimensional bordism 2-category [arXiv:1411.0945]).Symmetry, as formalized by group theory, is ubiquitous across mathematics and science. Classical examples include point groups in crystallography, Noether's theorem relating differentiable symmetries and conserved quantities, and the classification of fundamental particles according to irreducible representations of the Poincaré group and the internal symmetry groups of the standard model. However, in some quantum settings, the notion of a group is no longer enough to capture all symmetries. Important motivating examples include Galois-like symmetries of von Neumann algebras, anyonic particles in condensed matter physics, and deformations of universal enveloping algebras. The language of tensor categories provides a unified framework to discuss these notions of quantum symmetry.Updated on Oct 20, 2023 04:02 PM PDT
The study of tensor categories involves the interplay of representation theory, combinatorics, number theory, and low dimensional topology (from a string diagram calculation, describing the 3-dimensional bordism 2-category [arXiv:1411.0945]).Symmetry, as formalized by group theory, is ubiquitous across mathematics and science. Classical examples include point groups in crystallography, Noether's theorem relating differentiable symmetries and conserved quantities, and the classification of fundamental particles according to irreducible representations of the Poincaré group and the internal symmetry groups of the standard model. However, in some quantum settings, the notion of a group is no longer enough to capture all symmetries. Important motivating examples include Galois-like symmetries of von Neumann algebras, anyonic particles in condensed matter physics, and deformations of universal enveloping algebras. The language of tensor categories provides a unified framework to discuss these notions of quantum symmetry.Updated on Oct 20, 2023 04:02 PM PDT -
Program Higher Categories and Categorification
Organizers: David Ayala (Montana State University), Clark Barwick (University of Edinburgh), David Nadler (University of California, Berkeley), LEAD Emily Riehl (Johns Hopkins University), Marcy Robertson (University of Melbourne), Peter Teichner (Max-Planck-Institut für Mathematik), Dominic Verity (Macquarie University)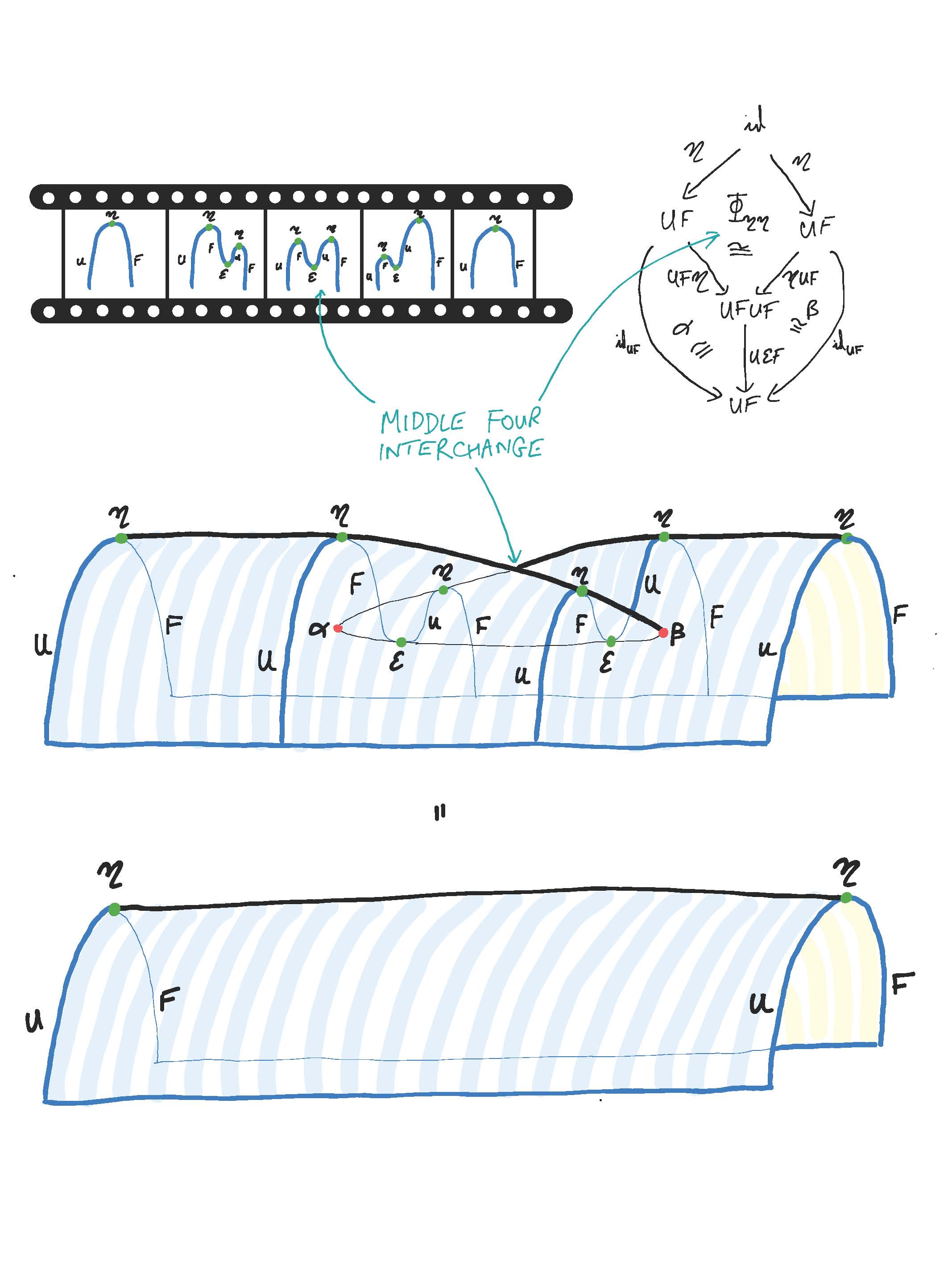 swallowtail identity
swallowtail identityThough many of the ideas in higher category theory find their origins in homotopy theory — for instance as expressed by Grothendieck’s “homotopy hypothesis” — the subject today interacts with a broad spectrum of areas of mathematical research. Unforeseen descent, or local-to-global formulas, for familiar objects can be articulated in terms of higher invertible morphisms. Compatible associative deformations of a sequence of maps of spaces, or derived schemes, can putatively be represented by higher categories, as Koszul duality for E_n-algebras suggests. Higher categories offer unforeseen characterizing universal properties for familiar constructions such as K-theory. Manifold theory is natively connected to higher category theory and adjunction data, a connection that is most famously articulated by the recently proven Cobordism Hypothesis.
In parallel, the idea of "categorification'' is playing an increasing role in algebraic geometry, representation theory, mathematical physics, and manifold theory, and higher categorical structures also appear in the very foundations of mathematics in the form of univalent foundations and homotopy type theory. A central mission of this semester will be to mitigate the exorbitantly high "cost of admission'' for mathematicians in other areas of research who aim to apply higher categorical technology and to create opportunities for potent collaborations between mathematicians from these different fields and experts from within higher category theory.Updated on Oct 20, 2023 12:44 PM PDT -
Program Complementary Program 2019-20
The Complementary Program has a limited number of memberships that are open to mathematicians whose interests are not closely related to the core programs; special consideration is given to mathematicians who are partners of an invited member of a core program.
Updated on Nov 27, 2018 12:28 PM PST -
Program Holomorphic Differentials in Mathematics and Physics
Organizers: LEAD Jayadev Athreya (University of Illinois at Urbana-Champaign), Steven Bradlow (University of Illinois at Urbana-Champaign), Sergei Gukov (California Institute of Technology), Andrew Neitzke (Yale University), Anna Wienhard (Max Planck Institute for Mathematics in the Sciences), Anton Zorich (Institut de Mathematiques de Jussieu)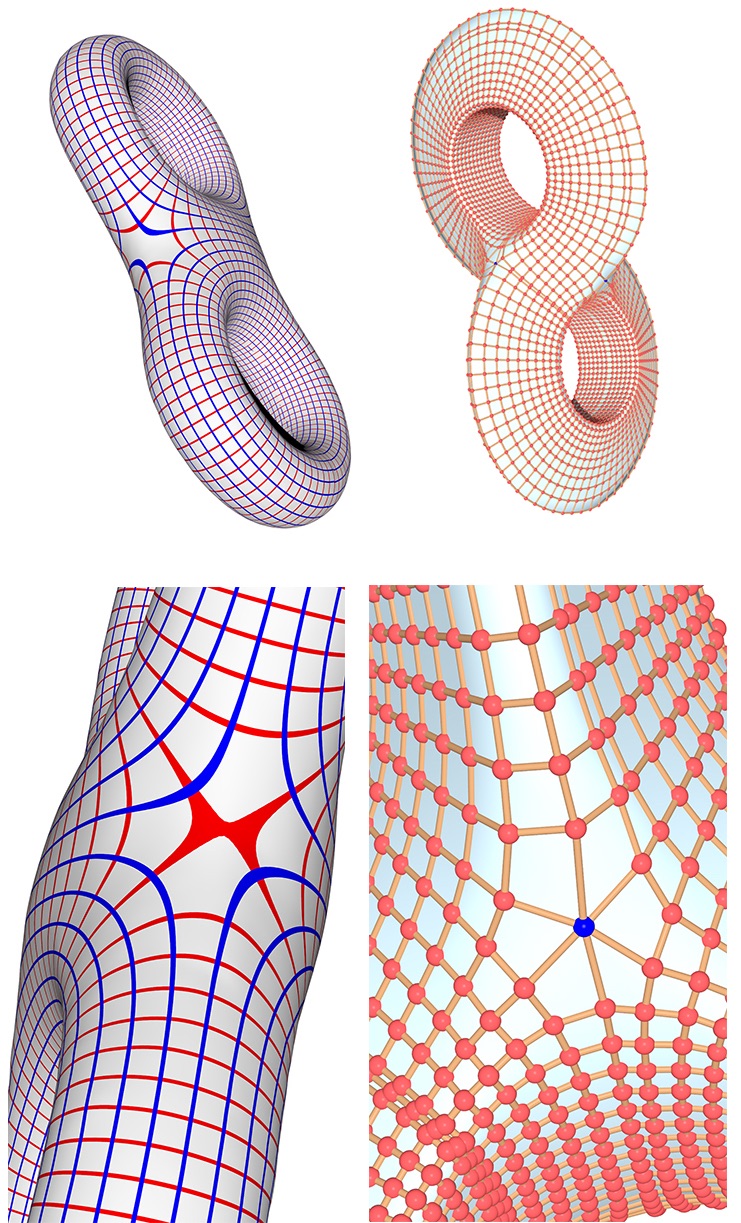 Some holomorphic differentials on a genus 2 surface, with close up views of singular points, image courtesy Jian Jiang.
Some holomorphic differentials on a genus 2 surface, with close up views of singular points, image courtesy Jian Jiang.Holomorphic differentials on Riemann surfaces have long held a distinguished place in low dimensional geometry, dynamics and representation theory. Recently it has become apparent that they constitute a common feature of several other highly active areas of current research in mathematics and also at the interface with physics. In some cases the areas themselves (such as stability conditions on Fukaya-type categories, links to quantum integrable systems, or the physically derived construction of so-called spectral networks) are new, while in others the novelty lies more in the role of the holomorphic differentials (for example in the study of billiards in polygons, special - Hitchin or higher Teichmuller - components of representation varieties, asymptotic properties of Higgs bundle moduli spaces, or in new interactions with algebraic geometry).
It is remarkable how widely scattered are the motivating questions in these areas, and how diverse are the backgrounds of the researchers pursuing them. Bringing together experts in this wide variety of fields to explore common interests and discover unexpected connections is the main goal of our program. Our program will be of interest to those working in many different elds, including low-dimensional dynamical systems (via the connection to billiards); differential geometry (Higgs bundles and related moduli spaces); and different types of theoretical physics (electron transport and supersymmetric quantum field theory).
Updated on Oct 20, 2023 04:13 PM PDT -
Program Microlocal Analysis
Organizers: Pierre Albin (University of Illinois at Urbana-Champaign), Nalini Anantharaman (Université de Strasbourg), Kiril Datchev (Purdue University), Raluca Felea (Rochester Institute of Technology), Colin Guillarmou (Université Paris-Saclay), LEAD Andras Vasy (Stanford University)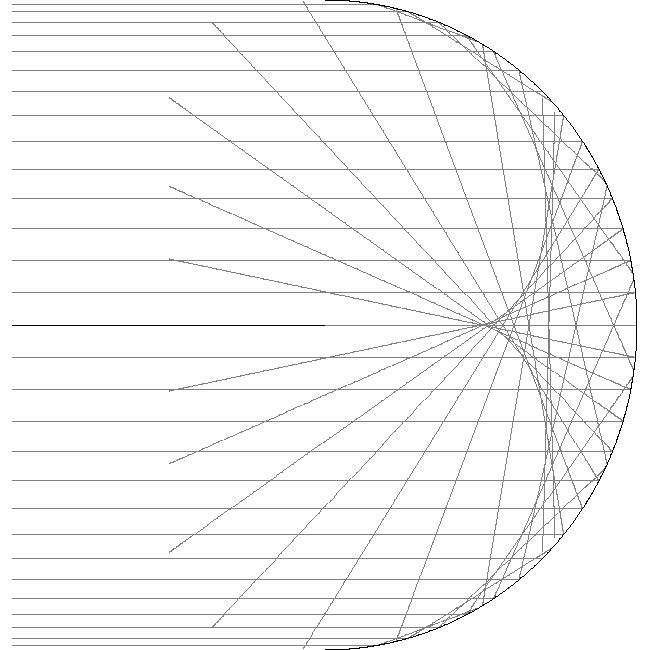
Microlocal analysis provides tools for the precise analysis of problems arising in areas such as partial differential equations or integral geometry by working in the phase space, i.e. the cotangent bundle, of the underlying manifold. It has origins in areas such as quantum mechanics and hyperbolic equations, in addition to the development of a general PDE theory, and has expanded tremendously over the last 40 years to the analysis of singular spaces, integral geometry, nonlinear equations, scattering theory… This program will bring together researchers from various parts of the field to facilitate the transfer of ideas, and will also provide a comprehensive introduction to the field for postdocs and graduate students.
Updated on Apr 13, 2018 11:42 AM PDT -
Program 2019 ADJOINT Program
Updated on Jan 28, 2025 05:03 PM PST -
Program Complementary Program 2018-19
The Complementary Program has a limited number of memberships that are open to mathematicians whose interests are not closely related to the core programs; special consideration is given to mathematicians who are partners of an invited member of a core program.
Updated on Jun 03, 2019 10:25 AM PDT -
Program Derived Algebraic Geometry
Organizers: Julie Bergner (University of Virginia), LEAD Bhargav Bhatt (Institute for Advanced Study), Dennis Gaitsgory (Harvard University), David Nadler (University of California, Berkeley), Nick Rozenblyum (University of Toronto), Peter Scholze (Universität Bonn), Gabriele Vezzosi (Università di Firenze) Courtesy of G. Karapet
Courtesy of G. KarapetDerived algebraic geometry is an extension of algebraic geometry that provides a convenient framework for directly treating non-generic geometric situations (such as non-transverse intersections in intersection theory), in lieu of the more traditional perturbative approaches (such as the “moving” lemma). This direct approach, in addition to being conceptually satisfying, has the distinct advantage of preserving the symmetries of the situation, which makes it much more applicable. In particular, in recent years, such techniques have found applications in diverse areas of mathematics, ranging from arithmetic geometry, mathematical physics, geometric representation theory, and homotopy theory. This semester long program will be dedicated to exploring these directions further, and finding new connections.
Updated on Oct 20, 2023 04:27 PM PDT -
Program Birational Geometry and Moduli Spaces
Organizers: Antonella Grassi (University of Pennsylvania), LEAD Christopher Hacon (University of Utah), Sándor Kovács (University of Washington), Mircea Mustaţă (University of Michigan), Martin Olsson (University of California, Berkeley)Birational Geometry and Moduli Spaces are two important areas of Algebraic Geometry that have recently witnessed a flurry of activity and substantial progress on many fundamental open questions. In this program we aim to bring together key researchers in these and related areas to highlight the recent exciting progress and to explore future avenues of research.This program will focus on the following themes: Geometry and Derived Categories, Birational Algebraic Geometry, Moduli Spaces of Stable Varieties, Geometry in Characteristic p>0, and Applications of Algebraic Geometry: Elliptic Fibrations of Calabi-Yau Varieties in Geometry, Arithmetic and the Physics of String TheoryUpdated on Jan 31, 2017 07:46 PM PST -
Program Hamiltonian systems, from topology to applications through analysis
Organizers: Rafael de la Llave (Georgia Institute of Technology), LEAD Albert Fathi (Georgia Institute of Technology; École Normale Supérieure de Lyon), vadim kaloshin (University of Maryland), Robert Littlejohn (University of California, Berkeley), Philip Morrison (University of Texas, Austin), Tere Seara (Polytechnical University of Cataluña (Barcelona)), Sergei Tabachnikov (Pennsylvania State University), Amie Wilkinson (University of Chicago)The interdisciplinary nature of Hamiltonian systems is deeply ingrained in its history. Therefore the program will bring together the communities of mathematicians with the community of practitioners, mainly engineers, physicists, and theoretical chemists who use Hamiltonian systems daily. The program will cover not only the mathematical aspects of Hamiltonian systems but also their applications, mainly in space mechanics, physics and chemistry.
The mathematical aspects comprise celestial mechanics, variational methods, relations with PDE, Arnold diffusion and computation. The applications concern celestial mechanics, astrodynamics, motion of satellites, plasma physics, accelerator physics, theoretical chemistry, and atomic physics.
The goal of the program is to bring to the forefront both the theoretical aspects and the applications, by making available for applications the latest theoretical developments, and also by nurturing the theoretical mathematical aspects with new problems that come from concrete problems of applications.
Updated on Aug 20, 2018 08:16 AM PDT -
Program Summer Research for Women in Mathematics
Organizers: Hélène Barcelo (MSRI / Simons Laufer Mathematical Sciences Institute (SLMath))See this LINK for the 2019 Summer Research for Women in Mathematics program.The purpose of the MSRI's program, Summer Research for Women in Mathematics, is to provide space and funds to groups of women mathematicians to work on a research project at MSRI. Research projects can arise from work initiated at a Women's Conference, or can be freestanding activities.Updated on Sep 11, 2018 01:32 PM PDT -
Program Complementary Program 2017-18
Updated on Nov 30, 2017 03:30 PM PST -
Program Group Representation Theory and Applications
Organizers: Robert Guralnick (University of Southern California), Alexander Kleshchev (University of Oregon), Gunter Malle (Universität Kaiserslautern), Gabriel Navarro (University of Valencia), Julia Pevtsova (University of Washington), Raphael Rouquier (University of California, Los Angeles), LEAD Pham Tiep (Rutgers University)Group Representation Theory is a central area of Algebra, with important and deep connections to areas as varied as topology, algebraic geometry, number theory, Lie theory, homological algebra, and mathematical physics. Born more than a century ago, the area still abounds with basic problems and fundamental conjectures, some of which have been open for over five decades. Very recent breakthroughs have led to the hope that some of these conjectures can finally be settled. In turn, recent results in group representation theory have helped achieve substantial progress in a vast number of applications.
The goal of the program is to investigate all these deep problems and the wealth of new results and directions, to obtain major progress in the area, and to explore further applications of group representation theory to other branches of mathematics.
Updated on Jan 12, 2018 04:00 PM PST -
Program Enumerative Geometry Beyond Numbers
Organizers: Mina Aganagic (University of California, Berkeley), Denis Auroux (University of California, Berkeley), Jim Bryan (University of British Columbia), LEAD Andrei Okounkov (Columbia University; University of California, Berkeley), Balazs Szendroi (University of Vienna)Traditional enumerative geometry asks certain questions to which the expected answer is a number: for instance, the number of lines incident with two points in the plane (1, Euclid), or the number of twisted cubic curves on a quintic threefold (317 206 375). It has however been recognized for some time that the numerics is often just the tip of the iceberg: a deeper exploration reveals interesting geometric, topological, representation-, or knot-theoretic structures. This semester-long program will be devoted to these hidden structures behind enumerative invariants, concentrating on the core fields where these questions start: algebraic and symplectic geometry.
Updated on Jan 16, 2018 10:12 AM PST -
Program Geometric Functional Analysis and Applications
Organizers: Franck Barthe (Université de Toulouse III (Paul Sabatier)), Marianna Csornyei (University of Chicago), Boaz Klartag (Weizmann Institute of Science), Alexander Koldobsky (University of Missouri), Rafal Latala (University of Warsaw), LEAD Mark Rudelson (University of Michigan)Geometric functional analysis lies at the interface of convex geometry, functional analysis and probability. It has numerous applications ranging from geometry of numbers and random matrices in pure mathematics to geometric tomography and signal processing in engineering and numerical optimization and learning theory in computer science.
One of the directions of the program is classical convex geometry, with emphasis on connections with geometric tomography, the study of geometric properties of convex bodies based on information about their sections and projections. Methods of harmonic analysis play an important role here. A closely related direction is asymptotic geometric analysis studying geometric properties of high dimensional objects and normed spaces, especially asymptotics of their quantitative parameters as dimension tends to infinity. The main tools here are concentration of measure and related probabilistic results. Ideas developed in geometric functional analysis have led to progress in several areas of applied mathematics and computer science, including compressed sensing and random matrix methods. These applications as well as the problems coming from computer science will be also emphasised in our program.
Updated on Aug 23, 2017 03:38 PM PDT -
Program Geometric and Topological Combinatorics
Organizers: Jesus De Loera (University of California, Davis), Victor Reiner (University of Minnesota Twin Cities), LEAD Francisco Santos Leal (University of Cantabria), Francis Su (Harvey Mudd College), Rekha Thomas (University of Washington), Günter Ziegler (Freie Universität Berlin)Combinatorics is one of the fastest growing areas in contemporary Mathematics, and much of this growth is due to the connections and interactions with other areas of Mathematics. This program is devoted to the very vibrant and active area of interaction between Combinatorics with Geometry and Topology. That is, we focus on (1) the study of the combinatorial properties or structure of geometric and topological objects and (2) the development of geometric and topological techniques to answer combinatorial problems.
Key examples of geometric objects with intricate combinatorial structure are point configurations and matroids, hyperplane and subspace arrangements, polytopes and polyhedra, lattices, convex bodies, and sphere packings. Examples of topology in action answering combinatorial challenges are the by now classical Lovász’s solution of the Kneser conjecture, which yielded functorial approaches to graph coloring, and the more recent, extensive topological machinery leading to breakthroughs on Tverberg-type problems.Updated on Aug 28, 2017 11:26 AM PDT -
Program Summer Research 2017
Come spend time at MSRI in the summer! The Institute’s summer graduate schools and undergraduate program fill the lecture halls and some of the offices, but we have room for a modest number of visitors to come to do research singly or in small groups, while enjoying the excellent mathematical facilities, the great cultural opportunities of Berkeley, San Francisco and the Bay area, the gorgeous natural surroundings, and the cool weather.
We can provide offices, library facilities and bus passes—unfortunately not financial support. Though the auditoria are largely occupied, there are blackboards and ends of halls, so 2-6 people could comfortably collaborate with one another. We especially encourage such groups to apply together.
To make visits productive, we require at least a two-week commitment. We strive for a wide mix of people, being sure to give special consideration to women, under-represented groups, and researchers from non-research universities.
Updated on May 31, 2018 12:40 PM PDT -
Program Complementary Program (2016-17)
The Complementary Program has a limited number of memberships that are open to mathematicians whose interests are not closely related to the core programs; special consideration is given to mathematicians who are partners of an invited member of a core program.
Updated on Apr 14, 2017 10:04 AM PDT -
Program Analytic Number Theory
Organizers: Chantal David (Concordia University), Andrew Granville (Université de Montréal), Emmanuel Kowalski (ETH Zurich), Philippe Michel (École Polytechnique Fédérale de Lausanne (EPFL)), Kannan Soundararajan (Stanford University), LEAD Terence Tao (University of California, Los Angeles)Analytic number theory, and its applications and interactions, are currently experiencing intensive progress, in sometimes unexpected directions. In recent years, many important classical questions have seen spectacular advances based on new techniques; conversely, methods developed in analytic number theory have led to the solution of striking problems in other fields.
This program will not only give the leading researchers in the area further opportunities to work together, but more importantly give young people the occasion to learn about these topics, and to give them the tools to achieve the next breakthroughs.
Updated on Jul 10, 2015 03:54 PM PDT -
Program Harmonic Analysis
Organizers: LEAD Michael Christ (University of California, Berkeley), Allan Greenleaf (University of Rochester), Steven Hofmann (University of Missouri), LEAD Michael Lacey (Georgia Institute of Technology), Svitlana Mayboroda (University of Minnesota, Twin Cities), Betsy Stovall (University of Wisconsin-Madison), Brian Street (University of Wisconsin-Madison)The field of Harmonic Analysis dates back to the 19th century, and has its roots in the study of the decomposition of functions using Fourier series and the Fourier transform. In recent decades, the subject has undergone a rapid diversification and expansion, though the decomposition of functions and operators into simpler parts remains a central tool and theme.This program will bring together researchers representing the breadth of modern Harmonic Analysis and will seek to capitalize on and continue recent progress in four major directions:-Restriction, Kakeya, and Geometric Incidence Problems-Analysis on Nonhomogeneous Spaces-Weighted Norm Inequalities-Quantitative Rectifiability and Elliptic PDE.Many of these areas draw techniques from or have applications to other fields of mathematics, such as analytic number theory, partial differential equations, combinatorics, and geometric measure theory. In particular, we expect a lively interaction with the concurrent program.Updated on Aug 11, 2016 10:49 AM PDT -
Program Geometric Group Theory
Organizers: Ian Agol (University of California, Berkeley), Mladen Bestvina (University of Utah), Cornelia Drutu (University of Oxford), LEAD Mark Feighn (Rutgers University), Michah Sageev (Technion---Israel Institute of Technology), Karen Vogtmann (University of Warwick)The field of geometric group theory emerged from Gromov’s insight that even mathematical objects such as groups, which are defined completely in algebraic terms, can be profitably viewed as geometric objects and studied with geometric techniques Contemporary geometric group theory has broadened its scope considerably, but retains this basic philosophy of reformulating in geometric terms problems from diverse areas of mathematics and then solving them with a variety of tools. The growing list of areas where this general approach has been successful includes low-dimensional topology, the theory of manifolds, algebraic topology, complex dynamics, combinatorial group theory, algebra, logic, the study of various classical families of groups, Riemannian geometry and representation theory.
The goals of this MSRI program are to bring together people from the various branches of the field in order to consolidate recent progress, chart new directions, and train the next generation of geometric group theorists.Updated on Aug 11, 2016 08:44 AM PDT -
Program Summer Research 2016
Come spend time at MSRI in the summer! The Institute’s summer graduate schools and undergraduate program fill the lecture halls and some of the offices, but we have room for a modest number of visitors to come to do research singly or in small groups, while enjoying the excellent mathematical facilities, the great cultural opportunities of Berkeley, San Francisco and the Bay area, the gorgeous natural surroundings, and the cool weather.
We can provide offices, library facilities and bus passes—unfortunately not financial support. Though the auditorium is largely occupied, there are blackboards throughout the institute, so 2-6 people can comfortably collaborate with one another. You are interested? Please apply, as a group, to our Summer Research program.
To make visits productive, we require at least a two-week commitment. We strive for a wide mix of people, being sure to give special consideration to women, under-represented groups, and researchers from non-research universities.
Updated on Aug 07, 2023 07:34 AM PDT -
Program Complementary Program
Updated on Jul 13, 2016 09:06 AM PDT -
Program Differential Geometry
Organizers: Tobias Colding (Massachusetts Institute of Technology), Simon Donaldson (Imperial College, London), John Lott (University of California, Berkeley), Natasa Sesum (Rutgers University), Gang Tian (Princeton University), LEAD Jeff Viaclovsky (University of Wisconsin-Madison)Differential geometry is a subject with both deep roots and recent advances. Many old problems in the field have recently been solved, such as the Poincaré and geometrization conjectures by Perelman, the quarter pinching conjecture by Brendle-Schoen, the Lawson Conjecture by Brendle, and the Willmore Conjecture by Marques-Neves. The solutions of these problems have introduced a wealth of new techniques into the field. This semester-long program will focus on the following main themes:
(1) Einstein metrics and generalizations,
(2) Complex differential geometry,
(3) Spaces with curvature bounded from below,
(4) Geometric flows,
and particularly on the deep connections between these areas.Updated on Oct 17, 2019 02:16 PM PDT -
Program New Challenges in PDE: Deterministic Dynamics and Randomness in High and Infinite Dimensional Systems
Organizers: Kay Kirkpatrick (University of Illinois at Urbana-Champaign), Yvan Martel (École Polytechnique), Jonathan Mattingly (Duke University), Andrea Nahmod (University of Massachusetts, Amherst), Pierre Raphael (Université Nice Sophia-Antipolis), Luc Rey-Bellet (University of Massachusetts, Amherst), LEAD Gigliola Staffilani (Massachusetts Institute of Technology), Daniel Tataru (University of California, Berkeley)The fundamental aim of this program is to bring together a core group of mathematicians from the general communities of nonlinear dispersive and stochastic partial differential equations whose research contains an underlying and unifying problem: quantitatively analyzing the dynamics of solutions arising from the flows generated by deterministic and non-deterministic evolution differential equations, or dynamical evolution of large physical systems, and in various regimes.
In recent years there has been spectacular progress within both communities in the understanding of this common problem. The main efforts exercised, so far mostly in parallel, have generated an incredible number of deep results, that are not just beautiful mathematically, but are also important to understand the complex natural phenomena around us. Yet, many open questions and challenges remain ahead of us. Hosting the proposed program at MSRI would be the most effective venue to explore the specific questions at the core of the unifying theme and to have a focused and open exchange of ideas, connections and mathematical tools leading to potential new paradigms. This special program will undoubtedly produce new and fundamental results in both areas, and possibly be the start of a new generation of researchers comfortable on both languages.
Updated on Sep 15, 2015 05:25 PM PDT -
Program Summer Research
Come spend time at MSRI in the summer! The Institute’s summer graduate schools and undergraduate program fill the lecture halls and some of the offices, but we have room for a modest number of visitors to come to do research singly or in small groups, while enjoying the excellent mathematical facilities, the great cultural opportunities of Berkeley, San Francisco and the Bay area, the gorgeous natural surroundings, and the cool weather.
We can provide offices, library facilities and bus passes—unfortunately not financial support. Though the auditoria are largely occupied, there are blackboards and ends of halls, so 2-6 people could comfortably collaborate with one another. We especially encourage such groups to apply together.
To make visits productive, we require at least a two-week commitment. We strive for a wide mix of people, being sure to give special consideration to women, under-represented groups, and researchers from non-research universities.
Updated on May 06, 2015 11:36 AM PDT -
Program Geometric and Arithmetic Aspects of Homogeneous Dynamics
Organizers: Alireza Golsefidy (University of California, San Diego), LEAD Dmitry Kleinbock (Brandeis University), Elon Lindenstrauss (The Hebrew University of Jerusalem), Hee Oh (Yale University), Jean-François Quint (CNRS - Université de Montpellier)Homogeneous dynamics is the study of asymptotic properties of the action of subgroups of Lie groups on their homogeneous spaces. This includes many classical examples of dynamical systems, such as linear Anosov diffeomorphisms of tori and geodesic flows on negatively curved manifolds. This topic is related to many branches of mathematics, in particular, number theory and geometry. Some directions to be explored in this program include: measure rigidity of multidimensional diagonal groups; effectivization, sparse equidistribution and sieving; random walks, stationary measures and stiff actions; ergodic theory of thin groups; measure classification in positive characteristic. It is a companion program to “Dynamics on moduli spaces of geometric structures”.
Updated on Jan 12, 2015 10:58 AM PST -
Program Complementary Program (2014-15)
Updated on Feb 27, 2014 09:09 AM PST -
Program Dynamics on Moduli Spaces of Geometric Structures
Organizers: Richard Canary (University of Michigan), William Goldman (University of Maryland), François Labourie (Universite de Nice Sophia Antipolis), LEAD Howard Masur (University of Chicago), Anna Wienhard (Max Planck Institute for Mathematics in the Sciences)The program will focus on the deformation theory of geometric structures on manifolds, and the resulting geometry and dynamics. This subject is formally a subfield of differential geometry and topology, with a heavy infusion of Lie theory. Its richness stems from close relations to dynamical systems, algebraic geometry, representation theory, Lie theory, partial differential equations, number theory, and complex analysis.
Updated on Apr 03, 2015 01:06 PM PDT -
Program Geometric Representation Theory
Organizers: LEAD David Ben-Zvi (University of Texas, Austin), Ngô Bảo Châu (University of Chicago), Thomas Haines (University of Maryland), Florian Herzig (University of Toronto), Kevin McGerty (University of Oxford), David Nadler (University of California, Berkeley), Catharina Stroppel (Rheinische Friedrich-Wilhelms-Universität Bonn), Eva Viehmann (TU München)The fundamental aims of geometric representation theory are to uncover the deeper geometric and categorical structures underlying the familiar objects of representation theory and harmonic analysis, and to apply the resulting insights to the resolution of classical problems. One of the main sources of inspiration for the field is the Langlands philosophy, a vast nonabelian generalization of the Fourier transform of classical harmonic analysis, which serves as a visionary roadmap for the subject and places it at the heart of number theory. A primary goal of the proposed MSRI program is to explore the potential impact of geometric methods and ideas in the Langlands program by bringing together researchers working in the diverse areas impacted by the Langlands philosophy, with a particular emphasis on representation theory over local fields.
Another focus comes from theoretical physics, where new perspectives on the central objects of geometric representation theory arise in the study supersymmetric gauge theory, integrable systems and topological string theory. The impact of these ideas is only beginning to be absorbed and the program will provide a forum for their dissemination and development.
Updated on Oct 17, 2019 01:13 PM PDT -
Program New Geometric Methods in Number Theory and Automorphic Forms
Organizers: Pierre Colmez (Institut de Mathématiques de Jussieu), LEAD Wee Teck Gan (National University of Singapore), Michael Harris (Columbia University), Elena Mantovan (California Institute of Technology), Ariane Mézard (Institut de Mathématiques de Jussieu; École Normale Supérieure), Akshay Venkatesh (Institute for Advanced Study)The branches of number theory most directly related to the arithmetic of automorphic forms have seen much recent progress, with the resolution of many longstanding conjectures. These breakthroughs have largely been achieved by the discovery of new geometric techniques and insights. The goal of this program is to highlight new geometric structures and new questions of a geometric nature which seem most crucial for further development. In particular, the program will emphasize geometric questions arising in the study of Shimura varieties, the p-adic Langlands program, and periods of automorphic forms.
Updated on Oct 11, 2013 02:02 PM PDT -
Program Model Theory, Arithmetic Geometry and Number Theory
Organizers: Ehud Hrushovski (The Hebrew University of Jerusalem), François Loeser (Sorbonne Université), David Marker (University of Illinois, Chicago), Thomas Scanlon (University of California, Berkeley), Sergei Starchenko (University of Notre Dame), LEAD Carol Wood (Wesleyan University)The program aims to further the flourishing interaction between model theory and other parts of mathematics, especially number theory and arithmetic geometry. At present the model theoretical tools in use arise primarily from geometric stability theory and o-minimality. Current areas of lively interaction include motivic integration, valued fields, diophantine geometry, and algebraic dynamics.
Updated on May 01, 2019 02:07 PM PDT -
Program Algebraic Topology
Organizers: Vigleik Angeltveit (Australian National University), Andrew Blumberg (Columbia University), Gunnar Carlsson (Stanford University), Teena Gerhardt (Michigan State University), LEAD Michael Hill (University of Minnesota), Jacob Lurie (Harvard University)Algebraic topology touches almost every branch of modern mathematics. Algebra, geometry, topology, analysis, algebraic geometry, and number theory all influence and in turn are influenced by the methods of algebraic topology. The goals of this 2014 program at MSRI are:
Bring together algebraic topology researchers from all subdisciplines, reconnecting the pieces of the field
Identify the fundamental problems and goals in the field, uncovering the broader themes and connections
Connect young researchers with the field, broadening their perspective and introducing them to the myriad approaches and techniques.
Updated on Oct 17, 2019 01:05 PM PDT -
Program Mathematical General Relativity
Organizers: Yvonne Choquet-Bruhat, Piotr Chrusciel (University of Vienna), Gregory Galloway (University of Miami), Gerhard Huisken (Math. Forschungsinstitut Oberwolfach; Eberhard-Karls-Universität Tübingen), LEAD James Isenberg (University of Oregon), Sergiu Klainerman (Princeton University), Igor Rodnianski (Princeton University), Richard Schoen (University of California, Irvine)The study of Einstein's general relativistic gravitational field equation, which has for many years played a crucial role in the modeling of physical cosmology and astrophysical phenomena, is increasingly a source for interesting and challenging problems in geometric analysis and PDE. In nonlinear hyperbolic PDE theory, the problem of determining if the Kerr black hole is stable has sparked a flurry of activity, leading to outstanding progress in the study of scattering and asymptotic behavior of solutions of wave equations on black hole backgrounds. The spectacular recent results of Christodoulou on trapped surface formation have likewise stimulated important advances in hyperbolic PDE. At the same time, the study of initial data for Einstein's equation has generated a wide variety of challenging problems in Riemannian geometry and elliptic PDE theory. These include issues, such as the Penrose inequality, related to the asymptotically defined mass of an astrophysical systems, as well as questions concerning the construction of non constant mean curvature solutions of the Einstein constraint equations. This semester-long program aims to bring together researchers working in mathematical relativity, differential geometry, and PDE who wish to explore this rapidly growing area of mathematics.
Updated on May 01, 2019 01:14 PM PDT -
Program Optimal Transport: Geometry and Dynamics
Organizers: Luigi Ambrosio (Scuola Normale Superiore), Yann Brenier (École Polytechnique), Panagiota Daskalopoulos (Columbia University), Lawrence Evans (University of California, Berkeley), Alessio Figalli (University of Texas, Austin), Wilfrid Gangbo (University of California, Los Angeles), LEAD Robert McCann (University of Toronto), Felix Otto (Max-Planck-Institut für Mathematik in den Naturwissenschaften), Neil Trudinger (Australian National University)In the past two decades, the theory of optimal transportation has emerged as a fertile field of inquiry, and a diverse tool for exploring applications within and beyond mathematics. This transformation occurred partly because long-standing issues could finally be resolved, but also because unexpected connections emerged which linked these questions to classical problems in geometry, partial differential equations, nonlinear dynamics, natural sciences, design problems and economics. The aim of this program will be to gather experts in optimal transport and areas of potential application to catalyze new investigations, disseminate progress, and invigorate ongoing exploration.
Updated on Oct 17, 2019 01:04 PM PDT -
Program Noncommutative Algebraic Geometry and Representation Theory
Organizers: Mike Artin (Massachusetts Institute of Technology), Viktor Ginzburg (University of Chicago), Catharina Stroppel (Universität Bonn , Germany), Toby Stafford* (University of Manchester, United Kingdom), Michel Van den Bergh (Universiteit Hasselt, Belgium), Efim Zelmanov (University of California, San Diego)Over the last few decades noncommutative algebraic geometry (in its many forms) has become increasingly important, both within noncommutative algebra/representation theory, as well as having significant applications to algebraic geometry and other neighbouring areas. The goal of this program is to explore and expand upon these subjects and their interactions. Topics of particular interest include noncommutative projective algebraic geometry, noncommutative resolutions of (commutative or noncommutative) singularities,Calabi-Yau algebras, deformation theory and Poisson structures, as well as the interplay of these subjects with the algebras appearing in representation theory--like enveloping algebras, symplectic reflection algebras and the many guises of Hecke algebras.
Updated on May 06, 2013 04:21 PM PDT -
Program Commutative Algebra
Organizers: David Eisenbud* (University of California, Berkeley), Srikanth Iyengar (University of Nebraska), Ezra Miller (Duke University), Anurag Singh (University of Utah), and Karen Smith (University of Michigan)Commutative algebra was born in the 19th century from algebraic geometry, invariant theory, and number theory. Today it is a mature field with activity on many fronts.
The year-long program will highlight exciting recent developments in core areas such as free resolutions, homological and representation theoretic aspects, Rees algebras and integral closure, tight closure and singularities, and birational geometry. In addition, it will feature the important links to other areas such as algebraic topology, combinatorics, mathematical physics, noncommutative geometry, representation theory, singularity theory, and statistics. The program will reflect the wealth of interconnections suggested by these fields, and will introduce young researchers to these diverse areas.
New connections will be fostered through collaboration with the concurrent MSRI programs in Cluster Algebras (Fall 2012) and Noncommutative Algebraic Geometry and Representation Theory (Spring 2013).
For more detailed information about the program please see, http://www.math.utah.edu/ca/.
Updated on Aug 18, 2013 04:09 PM PDT -
Program Complementary Program 2012-13
Updated on May 21, 2013 12:44 PM PDT -
Program Cluster Algebras
Organizers: Sergey Fomin (University of Michigan), Bernhard Keller (Université Paris Diderot - Paris 7, France), Bernard Leclerc (Université de Caen Basse-Normandie, France), Alexander Vainshtein* (University of Haifa, Israel), Lauren Williams (University of California, Berkeley)Cluster algebras were conceived in the Spring of 2000 as a tool for studying dual canonical bases and total positivity in semisimple Lie groups. They are constructively defined commutative algebras with a distinguished set of generators (cluster variables) grouped into overlapping subsets (clusters) of fixed cardinality. Both the generators and the relations among them are not given from the outset, but are produced by an iterative process of successive mutations. Although this procedure appears counter-intuitive at first, it turns out to encode a surprisingly widespread range of phenomena, which might explain the explosive development of the subject in recent years.
Cluster algebras provide a unifying algebraic/combinatorial framework for a wide variety of phenomena in settings as diverse as quiver representations, Teichmueller theory, invariant theory, tropical calculus, Poisson geometry, Lie theory, and polyhedral combinatorics.
Updated on May 06, 2013 04:25 PM PDT -
Program Random Spatial Processes
Organizers: Mireille Bousquet-Mélou (Université de Bordeaux I, France), Richard Kenyon* (Brown University), Greg Lawler (University of Chicago), Andrei Okounkov (Columbia University), and Yuval Peres (Microsoft Research Laboratories)In recent years probability theory (and here we mean probability theory in the largest sense, comprising combinatorics, statistical mechanics, algorithms, simulation) has made immense progress in understanding the basic two-dimensional models of statistical mechanics and random surfaces. Prior to the 1990s the major interests and achievements of probability theory were (with some exceptions for dimensions 4 or more) with respect to one-dimensional objects: Brownian motion and stochastic processes, random trees, and the like. Inspired by work of physicists in the ’70s and ’80s on conformal invariance and field theories in two dimensions, a number of leading probabilists and combinatorialists began thinking about spatial process in two dimensions: percolation, polymers, dimer models, Ising models. Major breakthroughs by Kenyon, Schramm, Lawler, Werner, Smirnov, Sheffield, and others led to a rigorous underpinning of conformal invariance in two-dimensional systems and paved the way for a new era of “two-dimensional” probability theory.
Updated on Aug 10, 2015 02:30 PM PDT -
Program Quantitative Geometry
Organizers: Keith Ball (University College London, United Kingdom), Emmanuel Breuillard (Université Paris-Sud 11, France) , Jeff Cheeger (New York University, Courant Institute), Marianna Csornyei (University College London, United Kingdom), Mikhail Gromov (Courant Institute and Institut des Hautes Études Scientifiques, France), Bruce Kleiner (New York University, Courant Institute), Vincent Lafforgue (Université Pierre et Marie Curie, France), Manor Mendel (The Open University of Israel), Assaf Naor* (New York University, Courant Institute), Yuval Peres (Microsoft Research Laboratories), and Terence Tao (University of California, Los Angeles)The fall 2011 program "Quantitative Geometry" is devoted to the investigation of geometric questions in which quantitative/asymptotic considerations are inherent and necessary for the formulation of the problems being studied. Such topics arise naturally in a wide range of mathematical disciplines, with significant relevance both to the internal development of the respective fields, as well as to applications in areas such as theoretical computer science. Examples of areas that will be covered by the program are: geometric group theory, the theory of Lipschitz functions (e.g., Lipschitz extension problems and structural aspects such as quantitative differentiation), large scale and coarse geometry, embeddings of metric spaces and their applications to algorithm design, geometric aspects of harmonic analysis and probability, quantitative aspects of linear and non-linear Banach space theory, quantitative aspects of geometric measure theory and isoperimetry, and metric invariants arising from embedding theory and Riemannian geometry. The MSRI program aims to crystallize the interactions between researchers in various relevant fields who might have a lack of common language, even though they are working on related questions.
Updated on Oct 17, 2019 02:39 PM PDT -
Program Free Boundary Problems, Theory and Applications
Organizers: Luis Caffarelli (University of Texas, Austin), Henri Berestycki (Centre d'Analyse et de Mathématique Sociales, France), Laurence C. Evans (University of California, Berkeley), Mikhail Feldman (University of Wisconsin, Madison), John Ockendon (University of Oxford, United Kingdom), Arshak Petrosyan (Purdue University), Henrik Shahgholian* (The Royal Institute of Technology, Sweden), Tatiana Toro (University of Washington), and Nina Uraltseva (Steklov Mathematical Institute, Russia)This program aims at the study of various topics within the area of Free Boundaries Problems, from the viewpoints of theory and applications. Many problems in physics, industry, finance, biology, and other areas can be described by partial differential equations that exhibit apriori unknown sets, such as interfaces, moving boundaries, shocks, etc. The study of such sets, also known as free boundaries, often occupies a central position in such problems. The aim of this program is to gather experts in the field with knowledge of various applied and theoretical aspects of free boundary problems.
Updated on Oct 17, 2019 01:42 PM PDT -
Program Arithmetic Statistics
Organizers: Brian Conrey (American Institute of Mathematics), John Cremona (University of Warwick, United Kingdom), Barry Mazur (Harvard University), Michael Rubinstein* (University of Waterloo, Canada ), Peter Sarnak (Princeton University), Nina Snaith (University of Bristol, United Kingdom), and William Stein (University of Washington)L -functions attached to modular forms and/or to algebraic varieties and algebraic number fields are prominent in quite a wide range of number theoretic issues, and our recent growth of understanding of the analytic properties of L-functions has already lead to profound applications regarding among other things the statistics related to arithmetic problems. This program will emphasize statistical aspects of L-functions, modular forms, and associated arithmetic and algebraic objects from several different perspectives — theoretical, algorithmic, and experimental.
Updated on Oct 17, 2019 02:19 PM PDT -
Program Random Matrix Theory, Interacting Particle Systems and Integrable Systems
Organizers: Jinho Baik (University of Michigan), Alexei Borodin (California Institute of Technology), Percy A. Deift* (New York University, Courant Institute), Alice Guionnet (École Normale Supérieure de Lyon, France), Craig A. Tracy (University of California, Davis), and Pierre van Moerbeke, (Université Catholique de Louvain, Belgium)The goal of this program is to showcase the many remarkable developments that have taken place in the past decade in Random Matrix Theory (RMT) and to spur on further developments on RMT and the related areas Interacting Particle Systems (IPS) and Integrable Systems (IS): IPS provides an arena in which RMT behavior is frequently observed, and IS provides tools which are often useful in analyzing RMT and IPS/RMT behavior.
Updated on Oct 17, 2019 01:08 PM PDT -
Program Inverse Problems and Applications
Organizers: Liliana Borcea (Rice University), Maarten V. de Hoop (Purdue University), Carlos E. Kenig (University of Chicago), Peter Kuchment (Texas A&M University), Lassi Päivärinta (University of Helsinki, Finland), Gunther Uhlmann* (University of Washington), and Maciej Zworski (University of California, Berkeley)Inverse Problems are problems where causes for a desired or an observed effect are to be determined. They lie at the heart of scientific inquiry and technological development. Applications include a number of medical as
well as other imaging techniques, location of oil and mineral deposits in the earth's substructure, creation of astrophysical images from telescope data, finding cracks and interfaces within materials, shape optimization,
model identification in growth processes and, more recently, modelling in the life sciences. During the last 10 years or so there has been significant developments both in the mathematical theory and applications of inverse problems. The purpose of the program would be to bring together people working on different aspects of the field, to appraise the current status of development and to encourage interaction between mathematicians and scientists and engineers working directly with the applications.Updated on Aug 09, 2023 03:57 PM PDT -
Program Homology Theories of Knots and Links
Organizers: Mikhail Khovanov (Columbia University), Dusa McDuff (Barnard College), Peter Ozsváth* (Columbia University), Lev Rozansky (University of North Carolina), Peter Teichner (University of California, Berkeley), Dylan Thurston (Barnard College), and Zoltan Szabó (Princeton University)The aims of this program will be to achieve the following goals:
- Promote communication with related disciplines, including the symplectic geometry program in 2009-2010.
- Lead to new breakthroughs in the subject and find new applications to low dimensional topology (knot theory, three-manifold topology, and smooth four manifold topology).
- Educate a new generation of graduate students and PhD students in this exciting and rapidly-changing subject.
The program will focus on algebraic link homology and Heegaard Floer homology.
Updated on May 22, 2025 05:53 PM PDT -
Program Symplectic and Contact Geometry and Topology
Organizers: Yakov Eliashberg *(Stanford University), John Etnyre (Georgia Institute of Technology), Eleny-Nicoleta Ionel (Stanford University), Dusa McDuff (Barnard College), and Paul Seidel (Massachusetts Institute of Technology)In the slightly more than two decades that have elapsed since the fields of Symplectic and Contact Topology were created, the field has grown enormously and unforeseen new connections within Mathematics and Physics have been found. The goals of the 2009-10 program at MSRI are to:
I. Promote the cross-pollination of ideas between different areas of symplectic and contact geometry;
II. Help assess and formulate the main outstanding fundamental problems and directions in the field;
III. Lead to new breakthroughs and solutions of some of the main problems in the area;
IV. Discover new applications of symplectic and contact geometry in mathematics and physics;
V. Educate a new generation of young mathematicians, giving them a broader view of the subject and the capability to employ techniques from different areas in their research.
Updated on Apr 19, 2014 09:30 PM PDT -
Program Tropical Geometry
Organizers: Eva-Maria Feichtner *(University of Bremen), Ilia Itenberg (Institut de Recherche Mathématique Avancée de Strasbourg), Grigory Mikhalkin (Université de Genève), and Bernd Sturmfels (UCB - University of California, Berkeley)Tropical Geometry is the algebraic geometry over the min-plus algebra. It is a young subject that in recent years has both established itself as an area of its own right and unveiled its deep connections to numerous branches of pure and applied mathematics. From an algebraic geometric point of view, algebraic varieties over a field with non-archimedean valuation are replaced by polyhedral complexes, thereby retaining much of the information about the original varieties. From the point of view of complex geometry, the geometric combinatorial structure of tropical varieties is a maximal degeneration of a complex structure on a manifold.
The tropical transition from objects of algebraic geometry to the polyhedral realm is an extension of the classical theory of toric varieties. It opens problems on algebraic varieties to a completely new set of techniques, and has already led to remarkable results in Enumerative Algebraic Geometry, Dynamical Systems and Computational Algebra, among other fields, and to applications in Algebraic Statistics and Statistical Physics.
Updated on Jul 11, 2025 01:23 PM PDT -
Program Algebraic Geometry
Organizers: William Fulton (University of Michigan), Joe Harris (Harvard University), Brendan Hassett (Rice University), János Kollár (Princeton University), Sándor Kovács* (University of Washington), Robert Lazarsfeld (University of Michigan), and Ravi Vakil (Stanford University)Updated on May 22, 2025 05:53 PM PDT -
Program Analysis on Singular Spaces
Organizers: Gilles Carron (University of Nantes), Eugenie Hunsicker (Loughborough University), Richard Melrose (Massachusetts Institute of Technology), Michael Taylor (Andras VasyUniversity of North Carolina, Chapel Hill), and Jared Wunsch (Northwestern University)Updated on Jan 08, 2025 02:42 PM PST -
Program Ergodic Theory and Additive Combinatorics
Organizers: Ben Green (University of Cambridge), Bryna Kra (Northwestern University), Emmanuel Lesigne (University of Tours), Anthony Quas (University of Victoria), Mate Wierdl (University of Memphis)Updated on Jul 01, 2025 02:12 PM PDT -
Program Representation Theory of Finite Groups and Related Topics
Organizers: J. L. Alperin, M. Broue, J. F. Carlson, A. Kleshchev, J. Rickard, B. SrinivasanCurrent research centers on many open questions, i.e., representations over the integers or rings of positive characteristic, correspondence of characters and derived equivalences of blocks. Recently we have seen active interactions in group cohomology involving many areas of topology and algebra. The focus of this program will be on these areas with the goal of fostering emerging interdisciplinary connections among them.
Updated on Jul 25, 2025 03:22 PM PDT -
Program Combinatorial Representation Theory
Organizers: P. Diaconis, A. Kleshchev, B. Leclerc, P. Littelmann, A. Ram, A. Schilling, R. StanleyRecent catalysts stimulating growth of this field in the last few decades have been the discovery of "crystals" and the development of the combinatorics of affine Lie groups.. Today the subject intersects several fields: combinatorics, representation theory, analysis, algebraic geometry, Lie theory, and mathematical physics. The goal of this program is to bring together experts in these areas together in one interdisciplinary setting.
Updated on Jul 25, 2025 02:55 AM PDT -
Program Geometric Group Theory
Organizers: Mladen Bestvina, Jon McCammond, Michah Sageev, Karen VogtmannIn the 1980’s, attention to the geometric structures which cell complexes can carry shed light on earlier combinatorial and topological investigations into group theory, stimulating other provacative and innovative ideas over the past 20 years. As a consequence, geometric group theory has developed many different facets, including geometry, topology, analysis, logic.
Updated on Apr 23, 2025 01:46 PM PDT -
Program Teichmuller Theory and Kleinian Groups
Organizers: Jeffrey Brock, Richard Canary, Howard Masur, Maryam Mirzakhani, Alan ReidThese fields have each seen recent dramatic changes: new techniques developed, major conjectures solved, and new directions and connections forged. Yet progress has been made in parallel without the level of communication across these two fields that is warranted. This program will address the need to strengthen connections between these two fields, and reassess new directions for each.
Updated on Jul 15, 2025 10:46 AM PDT -
Program Dynamical Systems
Organizers: Christopher Jones, Jonathan Mattingly, Igor Mezic, Andrew Stuart, Lai-Sang YoungThis program will take place at the interface of the theory and applications of dynamical systems. The goal will be to assess the current state-of-the-art and define directions for future research. Mathematicians who are developing a new generation of ideas in dynamical systems will be brought together with researchers who are using the techniques of dynamical systems in applied areas. A wide range of applications will be considered through four contextual settings around which the program will be organized. Some of the areas of concentration have greater emphasis on extending existing ideas in dynamical systems theory, rendering them more suitable for applications. Others are more directed toward seeking out potential areas of applications in which dynamical systems is likely to have a bigger role to play.
The four themes that will mold the semester are: (1) Extended dynamical systems, (2) Stochastic dynamical systems, (3) Control theory, and (4) Computation and modeling. The introductory workshop, which will be held in mid-January, will emphasize extended dynamical systems that occur as high-dimensional systems, such as on lattices or as partial differential equations. There will be a workshop on stochastic systems and control theory in March. The last theme will pervade the semester through seminar and working group activities.Updated on May 01, 2025 01:23 PM PDT -
Program Geometric Evolution Equations and Related Topics
Organizers: Bennett Chow, Panagiota Daskalopoulos, Gerhardt Huisken, Peter Li, Lei Ni, Gang TianThe focus will be on geometric evolution equations, function theory and related elliptic and parabolic equations. Geometric flows have been applied to a variety of geometric, topological, analytical and physical problems. Linear and nonlinear elliptic and parabolic partial differential equations have been studied by continuous, discrete and computational methods. There are deep connections between the geometry and analysis of Riemannian and Kähler manifolds.
Updated on Jul 01, 2025 02:12 PM PDT -
Program Computational Applications of Algebraic Topology
Organizers: Gunnar Carlsson, Persi Diaconis, Susan Holmes, Rick Jardine, Günter M. ZieglerUpdated on Jul 22, 2024 11:35 AM PDT -
Program New Topological Structures in Physics
Organizers: M. Aganagic, R. Cohen, P. Horava, A. Klemm, J. Morava, H. Nakajima, Y. RuanThe interplay between quantum field theory and mathematics during the past several decades has led to new concepts of mathematics, which will be explored and developed in this program. This includes: Stringy topology, branes and orbifolds, Generalized McKay correspondences and representation theory and Gromov-Witten theory.
Updated on Jul 16, 2025 11:36 AM PDT -
Program Rational and Integral Points on Higher-Dimensional Varieties
Organizers: Fedor Bogomolov, Jean-Louis Colliot-Thélène, Bjorn Poonen, Alice Silverberg, Yuri TschinkelOur focus will be rational and integral points on varieties of dimension > 1. Recently it has become clear that many branches of mathematics can be brought to bear on problems in the area: complex algebraic geometry, Galois and 4etale cohomology, transcendence theory and diophantine approximation, harmonic analysis, automorphic forms, and analytic number theory. Sometimes it is only by combining techniques that progress is made. We will bring together researchers from these various fields who have an interest in arithmetic applications, as well as specialists in arithmetic geometry itself.
Updated on Apr 01, 2025 01:35 PM PDT -
Program Nonlinear Dispersive Equations
Organizers: Carlos Kenig, Sergiu Klainerman, Christophe Sogge, Gigliola Staffilani, Daniel TataruThe field of nonlinear dispersive equations has experienced a striking evolution over the last fifteen years. During that time many new ideas and techniques emerged, enabling one to work on problems which until quite recently seemed untouchable. The evolution process for this field has itsorigin in two ways of quantitatively measuring dispersion. One comes from harmonic analysis, which is used to establish certain dispersive (Lp) estimates for solutions to linear equations. The second has geometrical roots, namely in the analysis of vector fields generating the Lorentz groupassociated to the linear wave equation. Our semester program in nonlinear dispersive equations will bring together leading experts in both of these directions.
Updated on Jul 15, 2025 02:53 PM PDT -
Program Nonlinear Elliptic Equations and Its Applications
Organizers: Xavier Cabré, Luis Caffarelli, Lawrence C. Evans, Cristian Gutiérrez, Lihe Wang, Paul YangThe research in nonlinear elliptic equations is one of the most developed in Mathematics, and of great importance because of its interaction with other areas within Mathematics and for its applications in broader scientific disciplines such as fluid dynamics, phase transitions, mathematical finance and image processing in computer science.
Updated on Jul 22, 2025 09:55 AM PDT -
Program Probability, Algorithms and Statistical Physics
Organizers: Yuval Peres (co-chair), Alistair Sinclair (co-chair), David Aldous, Claire Kenyon, Harry Kesten, Jon Kleinberg, Fabio Martinelli, Alan Sokal, Peter Winkler, Uri ZwickUpdated on May 16, 2025 10:18 AM PDT -
Program Mathematical, Computational and Statistical Aspects of Image Analysis
Organizers: David Mumford (Brown University), Jitendra Malik (University of California, Berkeley), Donald Geman (John Hopkins University) and David Donoho (Stanford University)The field of image analysis is one of the newest and most active sources of inspiration for applied mathematics. Present day mathematical challenges in image analysis span a wide range of mathematical territory.
Updated on Dec 03, 2024 09:15 AM PST -
Program Hyperplane Arrangements and Application
Organizers: Michael Falk, Phil Hanlon, Toshitake Kohno, Peter Orlik, Alexander Varchenko, Sergey YuzvinskyThe theory of complex hyperplane arrangements has undergone tremendous growth since its beginnings thirty years ago in the work of Arnol'd, Breiskorn, Deligne, and Hattori. Connections with generalized hypergeometric functions, conformal field theory, representations of braid groups, and other areas have stimulated fascinating research into topology of arrangement complements. Topological research leads in turn to many new combinatorial and algebraic questions about arrangements.
Updated on Jul 01, 2025 02:11 PM PDT -
Program Differential Geometry
Organizers: Robert Bryant (co-chair), Frances Kirwan, Peter Petersen, Richard Schoen, Isadore Singer, and Gang Tian (co-chair)As classical as the subject is, it is currently undergoing a very vigorous development, interacting strongly with theoretical physics, mechanics, topology, algebraic geometry, partial differential equations, the calculus of variations, integrable systems, and many other subjects. The five main topics on which we propose to concentrate the program are areas that have shown considerable growth in the last ten years: Complex geometry, calibrated geometries and special holonomy; Geometric analysis; Symplectic geometry and gauge theory; Geometry and physics; Riemannian and metric geometry.
Updated on Jul 15, 2025 10:46 AM PDT -
Program Topological Aspects of Real Algebraic Geometry
Organizers: Selman Akbulut, Grisha Mikhalkin, Victoria Powers, Boris Shapiro, Frank Sottile (chair), and Oleg ViroThe topological approach to real algebraic geometry is due to Hilbert who realized the advantages of considering topological properties of real algebraic plane curves. Much progress on Hilbert's work was achieved in the 1970's by the schools of Rokhlin and Arnold, including new objects and questions on complexification and complex algebraic geometry, relation to piecewise linear geometry and combinatorics, and enumerative geometry. This continues today with new topics such as amoebas, new connections such as that with symplectic geometry, and new challenges such as those posed by real polynomial systems.
Updated on Jan 22, 2025 03:47 PM PST -
Program Discrete and Computational Geometry
Organizers: Jesús A. De Loera, Herbert Edelsbrunner, Jacob E. Goodman, János Pach, Micha Sharir, Emo Welzl, and Günter M. ZieglerDiscrete and Computational Geometry deals with the structure and complexity of discrete geometric objects as well with the design of efficient computer algorithms for their manipulation. This area is by its nature interdisciplinary and has relations to many other vital mathematical fields, such as algebraic geometry, topology, combinatorics, and probability theory; at the same time it is on the cutting edge of modern applications such as geographic information systems, mathematical programming, coding theory, solid modeling, and computational structural biology.
Updated on May 29, 2025 03:05 PM PDT -
Program Semi-Classical Analysis
Organizers: Robert Littlejohn, William H. Miller, Johannes Sjorstrand, Steven Zelditch, and Maciej ZworskiThe traditional mathematical study of semi-classical analysis has developed tremendously in the last thirty years following the introduction of microlocal analysis, that is local analysis in phase space, simultaneously in the space and Fourier transform variables. The purpose of this program is to bring together experts in traditional mathematical semi-classical analysis, in the new mathematics of "quantum chaos," and in physics and theoretical chemistry.
Updated on May 22, 2025 05:53 PM PDT -
Program Commutative Algebra
Organizers: Luchezar Avramov, Mark Green, Craig Huneke, Karen E. Smith and Bernd SturmfelsCommutative algebra comes from several sources, the 19th century theory of equations, number theory, invariant theory and algebraic geometry. The field has experienced a striking evolution over the last fifteen years. During that period the outlook of the subject has been altered, new connections to other areas have been established, and powerful techniques have been developed.
Updated on Jul 25, 2025 04:53 PM PDT -
Program Quantum Computation
Organizers: Dorit Aharonov, Charles Bennett, Richard Jozsa, Yuri Manin, Peter Shor, and Umesh Vazirani (chair)Quantum computation is an intellectually challenging and exciting area that touches on the foundations of both computer science and quantum physics.
Updated on Aug 08, 2024 10:58 AM PDT -
Program Infinite-Dimensional Algebras and Mathematical Physics
Organizers: E. Frenkel, V. Kac, I. Penkov, V. Serganova, G. ZuckermanThis program will discuss recent progress in the representation theory of infinite-dimensional algebras and superalgebras and their applications to other fields.
Updated on May 22, 2025 05:53 PM PDT -
Program Algebraic Stacks, Intersection Theory, and Non-Abelian Hodge Theory
Organizers: W. Fulton, L. Katzarkov, M. Kontsevich, Y. Manin,R. Pandharipande, T. Pantev, C. Simpson and A. VistoliAlgebraic stacks originally arose as solutions to moduli problems in which they were used to parametrize geometric objects in families.They have also arisen in studying homological properties of quotient singularities, non-abelian Hodge theory, string theory, etc. This program will focus on intersection theory on stacks, non-abelian Hodge theory and geometric n-stacks, perverse sheaves on stacks and the geometric Langlands program, D-brane charges in string theory, and moduli of gerbes and mirror symmetry.
Updated on Jan 08, 2025 01:09 PM PST -
Program Integral Geometry
Organizers: L. Barchini, S. Gindikin, A. Goncharov and J. WolfThis program will focus on recent advances in integral geometry,with a focus on theinterrelationships between integral geometry and the theory ofrepresentations (Penrosetransform in flag domains, horospherical transforms), complex geometry, symplectic geometry,algebraic analysis, and nonlinear differential equations.
There will be an Introductory Workshop in Inverse Problems and Integral Geometry August 13-24Updated on May 22, 2025 05:53 PM PDT -
Program Inverse Problems
Organizers: D. Colton, J. McLaughlin, W. Symes and G. UhlmannIn the last twenty years or so there have been substantial developments in the mathematical theory of inverse problems,and applications have arisen in many areas, ranging from geophysics to medical imaging to non-destructive evaluation of materials. The main topics of this program will be developments in inverse boundary value problems, and inverse scattering problems.
There will be an Introductory Workshop in Inverse Problems and Integral Geometry August 13-24Updated on Jan 08, 2025 02:42 PM PST -
Program Operator Algebras
Organizers: C. Anantharaman-Delaroche, H. Araki, A. Connes, J. Cuntz, E.G. Effros, U. Haagerup, V.F.R. Jones , M.A. Rieffel and D.V. VoiculescuThe noncommutative mathematics of operator algebras has grown in many directions and has made unexpected connections with other parts of mathematics and physics. Since the 1984-85 MSRI program in Operator Algebras, developments have continued at a rapid pace, and interactions with other fields such as elementary particle physics and quantum groups continue to grow.
Updated on Dec 24, 2024 08:27 PM PST -
Program Spectral Invariants
Organizers: Tom Branson, S.-Y. Alice Chang, Rafe Mazzeo and Kate OkikioluThe past few decades have witnessed many new developments in the broad area of spectral theory of geometric operators, centered around the study of new spectral invariants and their application to problems in conformal geometry, classification of 4-manifolds, index theory, relationship with scattering theory and other topics. This program will bring together people working on different problems in these areas.
Updated on May 22, 2025 05:53 PM PDT -
Program Algorithmic Number Theory
Organizers: Joe Buhler, Cynthia Dwork, Hendrik Lenstra Jr., Andrew Odlyzko, Bjorn Poonen and Noriko YuiNumber theorists have always made calculations, whether by hand, desk calculator, or computer. In recent years this predilection has extended in many directions, and has been reinforced by interest from other fields such as computer science, cryptography, and algebraic geometry. The Algorithmic Number Theory program at MSRI will cover these developments broadly, with an eye to making connections to some of these other areas.
Updated on May 22, 2025 05:53 PM PDT -
Program Noncommutative Algebra
Organizers: Michael Artin, Susan Montgomery, Claudio Procesi, Lance Small, Toby Stafford, Efim ZelmanovFor more information about this program, please see the original web page at:http://www.msri.org/activities/programs/9900/noncomm/index.html
Updated on Jul 18, 2025 05:23 PM PDT -
Program Numerical and Applied Mathematics
Organizers: Ivo Babuska, M. Vogelius, L. Wahlbin, R. Bank and D. ArnoldFor more information about this program, please see the original web page at:http://www.msri.org/activities/programs/9900/fem/index.html
Updated on Mar 03, 2025 01:01 PM PST -
Program Galois Groups and Fundamental Groups
Organizers: Eva Bayer, Michael Fried, David Harbater, Yasutaka Ihara, B. Heinrich Matzat, Michel Raynaud, John ThompsonFor more information about this event, please see the original web page at:http://www.msri.org/activities/programs/9900/galois/index.html
Updated on May 22, 2025 05:53 PM PDT -
Program Random Matrix Models and Their Applications
Organizers: Pavel Bleher (co-Chair), Alan Edelman, Alexander Its (co-Chair), Craig Tracy and Harold WidomFor more information about this program, please see the program's original web page at http://www.msri.org/activities/programs/9899/random/index.html
Updated on May 22, 2025 05:53 PM PDT -
Program Foundations of Computational Mathematics
Organizers: Felipe Cucker (co-Chair), Arieh Iserles (co-Chair), Tien Yien Li, Mike Overton, Jim Renegar, Mike Shub (co-Chair), Steve Smale, and Andrew StuartPlease see the program's webpage at http://www.msri.org/activities/programs/9899/focm/index.html for more information.
Updated on Mar 31, 2025 09:08 AM PDT -
Program Symbolic Computation in Geometry and Analysis
Organizers: Marie-Francoise Roy, Michael Singer (Chair) and Bernd SturmfelsPlease see the program webpage at http://www.msri.org/activities/programs/9899/symbcomp/index.html for more information.
Updated on May 22, 2025 05:53 PM PDT -
Program Model Theory of Fields
Organizers: Elisabeth Bouscaren, Lou van den Dries, Ehud Hrushovski, David Marker (co-Chair), Anand Pillay, Jose Felipe Voloch, and Carol Wood (co-Chair)Please see the program webpage at http://www.msri.org/activities/programs/9798/mtf/index.html for more information about this program.
Updated on Jan 23, 2025 11:29 AM PST -
Program Stochastic Analysis
Organizers: R. Banuelos, S. Evans, P. Fitzsimmons, E. Pardoux, D. Stroock, and R. WilliamsPlease see the program webpage at http://www.msri.org/activities/programs/9798/sa/index.html for more information.
Updated on Jul 21, 2025 04:52 PM PDT -
Program Harmonic Analysis
Organizers: Michael Christ, David Jerison, Carlos Kenig (Chair), Jill Pipher, and Elias Stein.Please see the program webpage at http://www.msri.org/activities/programs/9798/ha/index.html for more information.
Updated on Jul 15, 2025 02:53 PM PDT -
Program Low-dimensional Topology
Organizers: Joan Birman, Andrew Casson, Robion Kirby (Chair), and Ron SternPlease see the program webpage at http://www.msri.org/activities/programs/9697/ldt/index.html for more information.
Updated on Jul 21, 2025 03:45 PM PDT -
Program Combinatorics
Organizers: Louis Billera, Anders Bjorner, Curtis Greene, Rodica Simion, and Richard Stanley (Chair)Please see the program webpage at http://www.msri.org/activities/programs/9697/comb/index.html for more information.
Updated on Jul 01, 2025 02:11 PM PDT -
Program Convex Geometry and Geometric Functional Analysis
Organizers: Keith Ball, Eric Carlen, Erwin Lutwak, V. D. Milman, E. Odell, and N. Tomczak.Updated on May 16, 2025 09:08 AM PDT -
Program Several Complex Variables
Organizers: Jean-Pierre Demailly, Joseph J. Kohn, Junjiro Noguchi, Linda Rothschild, Michael Schneider, and Yum-Tong Siu (Chair)Please see the program webpage at http://www.msri.org/activities/programs/9596/scv/ for more information.
Updated on Aug 23, 2024 12:02 PM PDT -
Program Holomorphic Spaces
Organizers: Sheldon Axler (co-Chair), John McCarthy (co-Chair), Don Sarason (co-Chair), Joseph Ball, Nikolai Nikolskii, Mihai Putinar, and Cora SadoskyPlease see the program webpage at http://www.msri.org/activities/programs/9596/hs/ for more information.
Updated on Aug 23, 2024 12:02 PM PDT -
Program Complex Dynamics and Hyperbolic Geometry
Organizers: Bodil Branner, Steve Kerckhoff, Mikhail Lyubich, Curt McMullen (chair), and John SmillieUpdated on May 16, 2025 11:53 AM PDT -
Program Automorphic Forms
Organizers: Daniel Bump, Stephen Gelbart, Dennis Hejhal, Jeff Hoffstein (co-chairman), Steve Rallis (co-chairman), and Marie- France VignerasUpdated on May 22, 2025 05:53 PM PDT -
Program Dynamical Systems and Probabilistic Methods for PDE's
Organizers: Percy Deift (co-chairman), Philip Holmes, James Hyman, David Levermore, David McLaughlin (co-chairman), Clarence Eugene WayneUpdated on Jul 22, 2025 09:55 AM PDT -
Program Differential Geometry
Organizers: Werner Ballman, Raoul Bott, Carolyn Gordon, Mikhael Gromov, Karsten Grove, Blaine Lawson (chairman), Richard SchoenUpdated on Jul 16, 2025 11:36 AM PDT -
Program Transcendence and Diophantine Problems
Organizers: A. Baker (co-chairman), W. Brownawell, W. Schmidt (co- chairman), P. VojtaUpdated on Oct 06, 2023 10:02 AM PDT -
Program Algebraic Geometry
Organizers: E. Arbarello, A. Beauville, A. Beilinson, J. Harris, W. Fulton, J. Kollar, S. Mori, J. Steenbrink, H. Clemens & J. KollarUpdated on May 22, 2025 05:53 PM PDT -
Program Symbolic Dynamics
Organizers: R. Adler (chairman), J. Franks, D. Lind, S. WilliamsUpdated on Jan 26, 2025 10:14 AM PST -
Program Lie Groups and Ergodic Theory with Applications to Number Theory and Geometry
Organizers: H. Furstenberg, M. Ratner, P. Sarnak, R. Zimmer (chairman)Updated on Jul 15, 2025 10:46 AM PDT -
Program Statistics
Organizers: P. Bickel (chairman), L. LeCam, D. Siegmund, T. SpeedUpdated on Oct 06, 2023 10:02 AM PDT -
Program Strings in Mathematics and Physics
Organizers: O. Alvarez, D. Friedan, G. Moore, I.M. Singer (chairman), G. Segal, C. TaubesUpdated on Jul 16, 2025 11:36 AM PDT -
Program Partial Differential Equations and Continuum Mechanics
Organizers: L.C. Evans, A. Majda (chairman), G. Papanicolaou, T. SpencerUpdated on Jul 22, 2025 09:22 AM PDT -
Program Representations of Finite Groups
Organizers: J. Alperin, C. Curtis (chairman), W. Feit, P. FongUpdated on Apr 03, 2023 01:59 PM PDT -
Program Logic
Organizers: L. Harrington, A. Macintyre, D.A. Martin (chairman), R. ShoreUpdated on Jan 23, 2025 11:29 AM PST -
Program Algebraic Topology and its Applications
Organizers: R. Cohen (chairman), G. Carlsson, W.-C. Hsiang, J.D.S. JonesUpdated on Jul 16, 2025 11:36 AM PDT -
Program Combinatorial Group Theory and Geometry
Organizers: S. Adian, K. Brown, S. Gersten, J. Stallings (chairman)Updated on Mar 27, 2024 09:39 AM PDT -
Program Symplectic Geometry and Mechanics
Organizers: R. Devaney, V. Guillemin (co-chairman), H. Flaschka, A. Weinstein (co-chairman)Updated on May 22, 2025 05:53 PM PDT -
Program Classical Analysis
Organizers: C. Fefferman, E. Stein (chairman), G. WeissUpdated on May 22, 2025 05:53 PM PDT -
Program Representations of Lie Groups
Organizers: W. Schmid, D. Vogan, J. Wolf (chairman)Updated on Dec 03, 2024 09:26 AM PST -
Program Number Theory with Connections to Algebraic Geometry
Organizers: B. Gross (chairman), N. Katz, B. Mazur, K. Ribet, J. TateUpdated on Apr 01, 2025 01:35 PM PDT -
Program Geometric Function Theory
Organizers: D. Drasin, F. Gehring (chairman), I. Kra, A. MardenUpdated on Jan 12, 2024 04:25 PM PST -
Program Mathematical Economics
Organizers: K. Arrow, G. Debreu (chairman), A. Mas-ColellUpdated on Jul 20, 2021 10:09 AM PDT -
Program Computational Complexity
Organizers: R. Graham, R. Karp (co-chairman), S. Smale (co-chairman)Updated on May 22, 2025 05:53 PM PDT -
Program Differential Geometry
Organizers: S.-S. Chern (chairman), B. Lawson, I. M. Singer (miniprogram)Updated on May 22, 2025 05:53 PM PDT -
Program Low-dimensional Topology
Organizers: R. Edwards (chairman), R. Kirby, J. Morgan, W. ThurstonUpdated on Jan 02, 2025 09:54 AM PST -
Program K-Theory, Index Theory, and Operator Algebras
Organizers: A. Connes (chairman), R. Douglas, M. TakesakiUpdated on Mar 28, 2024 11:04 AM PDT -
Program Infinite-Dimensional Lie Algebras
Organizers: H. Garland, I. Kaplansky (chairman), B. KostantUpdated on Feb 05, 2024 03:44 PM PST -
Program Ergodic Theory and Dynamical Systems
Organizers: J. Feldman (chairman), J. Franks, A. Katok, J. Moser, R. TemamUpdated on Jun 09, 2023 11:07 AM PDT -
Program Mathematical Statistics
Organizers: L. LeCam, D. Siegmund (chairman), C. StoneUpdated on Dec 03, 2024 09:15 AM PST -
Program Nonlinear Partial Differential Equations
Organizers: A. Chorin, I. M. Singer (chairman), S.-T. YauUpdated on Jun 12, 2017 11:16 AM PDT
|
|
Past Programs |
